Week 2 Task 2: Substitution fractals
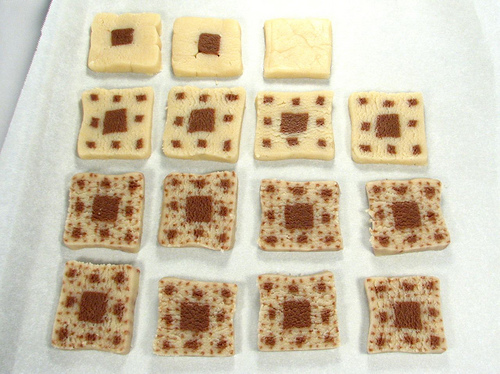
Fractal cookies by Evil Mad Scientist
Today your mission is…
Create your own substitution fractal and use it to explore multiplication.
Ready, Set, Go
Find a picture you like, with prominent parts inside its outline. The object can contain the parts, such as an owl’s head with two big eyes, or an open book with large illustrations. Or it can be made of the parts, for example, a word out of letters, or a window out of panels. You can also start with an abstract shape, like a triangle split into triangles.
Replace each part with a miniature copy of the whole shape. Repeat several times. You just drew several levels of a substitution fractal!
Respond to today's task
- What multiplication ideas do you see in this topic? How about ideas inspired by calculus?
- Did you use this with kids or students? How did it go? What did they say and do? What questions did they ask?
Help your child to get started
Some children can’t resist large-scale projects and making a substitution fractal is perfect for them. Get the largest piece of paper you can find and start big, with the base object taking up the entire sheet. Or take this activity outside where you can draw with chalk.
Draw a fractal based on a shape your child loves - a toy, a setting, an idea. Tell a story of what’s happening and encourage your child to add details to the story as you draw new levels of the fractal.
Toddlers
If your toddler likes stickers and collages, cut shapes out of different-color paper for different levels. Fold paper to cut several shapes at once. Or you can make fractal cookies out of real or play dough.
Young kids
Children realize early on that words are made out of letters. How about letters made out of words? Your kids can use this software to make math birthday cards for friends and relatives. http://www.edboas.com/science/ftext/index.html
Older kids
The study of fractals picked up in the late 1960s, with the use of computers. Tech literacy is essential for studying fractals beyond the first introduction to the idea. Make extreme and outlandish art with Droste Effect software: http://www.photospiralysis.com/
How is this multiplication?
Like tree fractals, substitution fractals support the foundations of our number system, but in a different way. Tree fractals show groups of groups of groups, for example, tens of tens of tens making a thousand. Substitution fractals can show that too, if you zoom out through their levels. But substitution fractals also show parts of parts of parts, such as one-tenth of one-tenth of one-tenth of a meter making a millimeter. This is one of the most systematic (and gorgeous) ways to explore multiples and fractions, positive and negative powers, multiplication and division within a single unifying pattern.
Inspired by calculus
Substitution fractals focus inward: you zoom in, almost like a microscope. They help children imagine not only infinitely large, but infinitely small, infinitesimal objects. It’s interesting to note that in a group, girls often prefer the subtlety and inward focus of substitution fractals, and boys often prefer the big expansion of tree fractals.
Frequently Asked Question
What if I can’t draw at all?!!
Babies don’t care if their parents finished their opera training before singing lullabies! However poorly you draw, kids will appreciate you drawing for them. But if you are anxious, start from simple geometric shapes, such as squares and triangles. You can also borrow someone’s math goggles, so to speak, to see how more complex objects come together from simple shapes. If all else fails, a circle with two triangles always makes a good cat portrait!
Words
Fractal, scale, power, exponent, recursion, zoom, reverse
Scavenger hunt
Matryoshka, by Radiology Art
Seek substitution fractals and nested pictures in art. They appear in abstract designs, such as nested dolls, but also in philosophical or symbolic art pieces, such as Bruegel’s “Big fish eat small fish.” Nested structures also appear in traditional architecture (check out these fractal villages in Africa), rhymes, and stories, such as “This is the house that Jack built.”
Fractal geometry will make you see everything differently. There is a danger in reading further. You risk the loss of your childhood vision of clouds, forests, flowers, galaxies, leaves, feathers, rocks, mountains, torrents of water, carpet, bricks, and much else besides. Never again will your interpretation of these things be quite the same.
- Michael Barnsley
Course links
- All course tasks
- Multiplication Lounge: our open forum for questions, comments, and ideas
- Course participants survey
ATTENDANCE: J (9), S (10), A (10), T (10) – all fourth grade homeschooled girls
SYNOPSIS OF ACTIVITIES
My tree fractal demonstration – I showed them a turtle pin that is a special piece of jewelry, and told them I’d like to do some math art in honor of it, and that I’d show them how and that I’d like their help. Then I started drawing. Several times, I asked them to pick up pencils and help but no one did until the fourth iteration, and then it was only S doing one turtle. As I worked, they brainstormed their own ideas.
As I was working on the 4th iteration, I started asking about/discussing the math behind this – why is this math, etc? We worked together on a chart of number patterns, and did some predicting. It was hard to describe which iteration we were talking about at first, since these kids had never worked with that concept (except possibly in science experiments a smidge). The kids suggested that we use real life vocabulary so we called the first turtle in the center “mom” then the next group “children” etc etc and this helped a lot and generated smiles.
Then the kids started working on their own. They attempted turtles, birds, suns, hearts, triangles, and infinity signs. As they worked challenges and questions arose, so we made running lists of “problems” and “questions” that we added to throughout class. (I’ll put the list at the end of the report.)
Photo I took a group photo now as the girls drew so that I would have it ready to use later on the computer.
Tree fractal software The kids played on the visnos.com site, and had a great time. They took turns at the computer, with lots of oohs and aahs and how did you do that’s. One student stayed late afterwards to do more. This was a fantastic way to introduce scale factor from a numerical perspective.
Fractal hand video They loved it, although found it creepy. We watched it 2-3 times. Used it to discuss substitution fractals (since the fingernails in this got replaced by smaller hands). I mentioned that I had seen one somewhere with an eye substituted for a mouth but can’t remember where. The kids want me to email that to them when I encounter it again (help?).
Substitution fractal I drew a quick example of this (a traffic light), and told the kids they could do this type too. I tried to get then to come up with the idea first by asking a bunch of questions about their frustrations with the tree fractal and large scale factors. I don’t think anyone ended up making their own substitution fractal, but some had ideas.
Letter fractals We played with letter fractals on the edboas site. That was fun. I encouraged kids to make their own by hand, but no one did. They were too taken by tree fractals. They loved the idea of using this site for birthday cards.
Photospiralysis They had fun with this using the photo of the 4 of them I took. I think everyone is looking forward to using these various sites at home.
Fractal scavenger hunt – did not do
Ted Talk on African Designs – did not show – I didn’t think that it would hold people’s interest b/c it didn’t hold mine (and I’ve tried to watch this several times over the past year or so). I think the topic is interesting but it needs to be presented in a different way for kids to appreciate it. (My guess is that you intended this for the adults only, but I wasn’t sure.) My issue, though, might also be that I’m overscheduled (like most working homeschooling parents) and it would take something even more spectacular to get me to watch a video for 16 minutes.
PROBLEMS (student-generated list)
- Keeping the shape consistent (with my turtle, sometimes the tail pointed right and sometimes left)
- Keeping size of shape consistent
- Running out of room for additional iterations (which generated nice discussion of adjusting scale factor)
- Having any room at all when doing a tree fractal with a scale factor bigger than one for a shape with width
- With shrinking iterations, drawing tiny things is hard
QUESTIONS (group collaboration; this list was still being added to even as people were walking out the door at the end)
- Will the points eventually connect?
- What’s the difference btwn a fractal and a tessellation?
- How many iterations/shapes could you draw if you had unlimited time, desire, and ability (robot? Computer? Magic?)
- What is the minimum number of points required on your shape to generate a fractal that’s not just a line?
- How do you know you’re finished?
- Is a hybrid tessellation/fractal possible? Has it been done? (This would have a scale factor, but no negative space.)
- If you connect each of your shapes of the second iteration to the first in a different way (i.e. connect one by its feet, another by its tail, etc), is it still a fractal? Is it still multiplication?
POST-CLASS REFLECTIONS
At one point when the kids were struggling with their tree fractals (how many points? curved lines or straight? is the scale factor sufficient for non-overlapping shapes?) I showed them my failed attempts from the night before. Seeing my failures made a huge difference in their confidence.
The above took about 1 hr 45 minutes. The kids then snacked and played with photospiralysis a bit in the dining room while two of the parents joined me in the living room for a bit of conversation. The parents were talking about how their kids do not feel confident in math (especially one who had spent two years in traditional public school). Their kids also do not like “school math” but enjoy “non-school math.” With this in mind, I called the kids back in to reflect on the session with the parents.
First I asked the girls what they would have named this course or session. (They knew I’d be giving feedback to you. Their proposals:
- The Family Tree Gathering (they had named the 4-generation turtle fractal “Our Family Tree”)
- Math Made Fun
- A Get Together Circle
- Math Get Together
- Fractals
Then I told them that you had actually already named this course, and that it was called “Multiplication.” Jaws dropped and eyes opened wide in shock. We had a nice discussion about how we had just been sitting around doing multiplication for a few hours.
Then I asked for specific comments about the session – what they liked, what they didn’t, and what they would change. They liked everything, but here are the things I jotted down as we discussed:
- Liked computer things
- More food (we scheduled this 9:30-11:30 so people were fading with hunger for lunch – I’ll ask the parents to bring more snacks next time)
- Play time afterwards (everyone had different activities and errands they needed to get to)
- Having dog here, but not interfering (my dog is laid back, calm, and quiet but likes to lie in the center of things. The kids liked petting her, but S did not like her trying to sneak the snacks. One parent stayed for the whole session and helped to manage the dog. We live in a tiny house, so dog management is a challenge. I like the kids to have the snacks on the floor while we do math to maximize the time we have to do the math, instead of taking a formal break, which causes this dog problem.)
- Having friends (S, the student who had been in public school – the social aspect was very important to her. Her mom told me that at her school, they had posted everyone’s test/quiz grades on the board to motivate kids – this was in kingergarten and first grade. So math for her was competitive.)
- Real life applications (one student, T, requested more of this)
- Science math (another recommendation from T)
- Integrating art (S stressed that having the art and having the dog really helped her to focus)
- More activities (things petered out after an hour and 45, but kids were hungry and parents were coming. I could have done the scavenger hunt, but didn’t.) I think they really really would have liked something else to draw by hand that was more different from the tree fractal task than was the substitution fractal.
- Recc. From J (with the others agreeing): integrate music and movement and acting and dance
- Recc from S (with others agreeing): a non-competitive game (brainstorming already began for this, so I promised them 10 minutes next time to brainstorm together – maybe they can have a 10 minute eat at the table break next time to do this away from the dog)
- A group fractal where each person did a different shape and put theirs in as a different iteration (T suggested this at the beginning, but everyone else wanted to do their own. By the time she suggested this again at the very end, the others were ready to collaborate but we were out of time)
Finally I asked them if there was anything that they didn’t like. J said that the first activity where I explained to them how to make the turtle fractal was not fun for her. She has grown up in exploratory math circles, so she really noticed that I was basically giving them an algorithm and asking them to execute it. That really cramped her style. Particularly when she had put her fractals aside to draw her favorite thing: interior design sketches. I asked her for today to try to stick with fractals since we had a goal here. (Later, she mentioned privately that it hurt her feelings when I asked her to do this.) The group did agree with her that they all would have preferred an activity that had them doing more thinking as opposed to following directions. I asked them, “Then how could you have known how to make a tree fractal?” They didn’t know. And of course 24 hours later with my 20/20 hindsight I can think of many ways I could have introduced this topic differently that would have involved some discovery on their part.
Rodi Steinig
PS Sorry this is so long. Maria, I tried to attach it as you suggested, but for some reason this file does not appear as a selection option when I click on the paperclip icon through this site. Weird.
We did this one and my 4yo daughter understood it much better than the one yesterday! She got the point really quickly and she said: "we are doing hearts inside hearts and we are getting tinny little ones! We can't go further because we don't hace a thinner pen!" So she really new we could go on and on... I love this activities!! I asked her how many hearts would she draw in the next turn and she counted the gaps before drawing them so she really understood it :)
I see how she understood dimension and space sharing, which appears to be divide by 2 in this case, isn't it?
Sweet, she got both the scale and the position of the next heart - all aspects of this, really! Including the idea that infinity lives in our imaginations, even if our pens are too thick to draw it.
We'll never know why substitution fractals made more sense, between the two. Maybe just because it's the second fractal activity in the family?
We have really been enjoying fractals. Yesterday we did tree fractals and Sieroinski triangles. We discussed the concept of exponents with these fractals and then went outside looking for examples in nature. Today both kids really enjoyed the substitution fractals, our five year old really loved drawing out the owl eye fractal. Our son drew his won tree fractals today and wrote out the exponents. We discussed the difference between 2+2+2+2=2x4 and 2x2x2x2. It was really fun.
OMG, my son is loving all of these fractals. He did his name on http://www.revisionrads.com/ftext/output/o18531.html and was blown away by how many times his name was written in each letter of his name. Very cool! On http://www.photospiralysis.com/WebAppGamma/RunApp.html (and with google search to help explain) we learned about the droste effect by playing with mirror, stretching, & zenith. He learned that daddy can do this with real pictures inside photoshop which lead to some funny business! We looked at images of Sierpinski Triangle Fractals (http://fractalfoundation.org/resources/fractivities/sierpinski-triangle/) and discussed how doodling like this is combining art and math.
