From buttons to the multiplication blind spots

Buttons as a a tangible representation of a set
My kids love playing with our button collection. Who does not? Buttons are colorful, cute, interesting objects. They are also an incredibly useful mathematical manipulative – a tangible representation of a set. Today we have decided to arrange 1, 2, 3, … , 34, 35, 36 buttons into groups. The first task was to divide the 36 buttons among three siblings.

36 is 3 times 12
Without the use of the multiplication table, sharing buttons is a game in its own right: “One for me, one for you, one for our toddler sister.” “I hate pink buttons. Let’s trade.” Surprisingly enough, every single one of them ended up with the exactly the same amount of buttons. So we went on to the next challenge. Can you guess what it was just from looking at the picture?

A new arrangement
One day we will arrange the buttons in a square and add the connection to 6 x 6.
In the good old (hold on, I am undecided on ‘good’, but I am definitely not that old) days, I learned the multiplication table with drills. No visualization, just tons of practice. I can still calculate the answers to mathematical problems in my head, on a piece of paper, or even on a blackboard when I teach. I also love talking to people about education and math teaching, in general. Some believe that today’s kids will live in a world surrounded by technology, thus we should teach them to type, program, and use a calculator as soon as possible. Others are horrified by today’s youth lacking mathematical skills. The rest are busy changing the educational landscape.
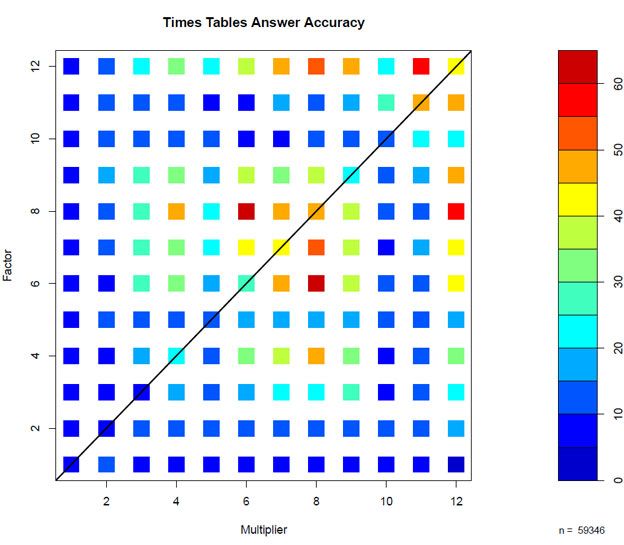
Recently, I have noticed that recalling 7 x 8 = 56 takes me a good deal longer than say, 8 x 3 = 24.
As a very curious person, I took a poll among my friends. Most of them have learned to multiply in a similar manner. :) To my great relief, I am not the only one suffering. Even though the location of the blind spots is individual. So far, I have just colored in the separate responses: 6×9, 7×8, and 8×7 seem to cause most trouble.

Multiplication blind spots
Can you recall all of the facts from the multiplication table equally well or do you suffer from a blind spot? If so, please share its location. I would love to create a more accurate map of multiplication table blind spots, but I need more respondents.
In the meanwhile, check out the Who, What, Why article Why does the sum 7×8 catch people out? about times tables answers accuracy.
Posted in Grow
Calculus for Kids April 19, 2015 (Sundays)
This is a story about the second meeting of a Calculus for Kids math circle. The math circle started off with the class attentively watching the trailer of Flatland. The movie itself is shown on a 2D screen, but it talks about journeys from 0 to 4 dimensions.



Download the Math Spark that invites you to travel to Flatland with us.
Today’s challenge is making 2D, flat shapes out of other shapes. Maddie and Sydney are discussing and examining how to transform Sydney’s green playdough shape into “what it is about to become” (they don’t know yet).

Sometimes, you just have to build something without even naming what it is. Yash built “something” imaginary out of LEGO blocks.

Maddie is making a 2D pair of glasses by coiling 1D pipe cleaners. She also created a 2D half-circle that transformed into a 3D sphere.

“Would you look at this!”


That flat object…

…Transforms into a sphere!

A triangle is not just a triangle, but a slice of pizza (as well as a 2D object).
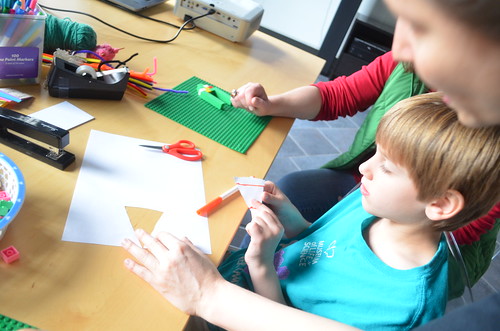
If you make several such shapes, you can arrange them into a whole pizza. Note how the children are building while listening. Doing something with your hands often helps to listen, even if it looks like children’s undivided attention is on their own project.

How do you know they are listening? Try asking a question: “What kind of shape would the slices of pizza make in a row instead of a circle?”

Hmm … let’s see!


(Spoiler: it makes a rectangle.)

Yash meanwhile finished creating another “something” – many sticky notes reminded grown-ups of the field of math called Chaos Theory. Maria asked him to write out his thoughts on the paper. In return he wrote: “I think it’s a bunch of sticky notes.”


Mark made a rectangle out of six smaller rectangle – order in contrast to chaos.

This rectangle, just like the chaotic 2D object Yash made, is abstract (not representing anything). The same technique can be used to model objects. On the other side of the table, Emma is discussing with Maria how to integrate a Minecraft sword out of sticky notes.

Hmm …


Nearly finished!

Mark is holding an imaginative 3D bow and arrow he made from LEGO blocks. It’s a flat shape that we can use as a 2D model.

Sydney is making an unknown shape. From blocks and imagination, she bravely creates something totally new. Older children and adults often lose that bravery and become hesitant to build things that don’t look like things. For Sydney, it’s the process of building that matters – and if the end product turns out interesting enough, like this tower, it’s good too, but not a requirement.

Eli just finished making a church. First, he integrated flat squares out of “dots” (LEGO blocks) and then he made the 3D building out of squares, one on top the other.

But instead, you can integrate “dots” into a 1D line – a really tall one, taller than you. But what if you got up on the chair?


Allison is making a 2D pattern inspired by a doll’s dress. Sydney is getting up to go make her own version of a sword (not shown), using a different grid layout. When children make objects out of rectangles (like sticky notes), different types of grids come up a lot.

We are about to watch and play with the String Spin interactive, and pose for a picture! “Make very silly faces for the New York Times!”


Mark is experimenting, drawing on the pad to see what kind of shape his string will create.

Sydney is predicting what will come of drawings.

There is a temptation to say everything looks like “a weird shape” – children use a generic word, maybe “alien” or “spaceship” for everything, instead of specifying a cone, a cylinder, or a sphere. But they are not wrong, they are just expressing the fact there are similarities in all the different shapes the toy makes. It’s a mathematical value to see similarities over differences.

You can use the “Yes, and…” improv technique here: “Yes, all these shape look like spaceships. And that’s because they are all figures of revolution, so they all are similar to one another!”
Photos by Erin Song, captions by Erin Song and Maria Droujkova, Math Spark by Kalid Azad, Shelley Nash, and Maria Droujkova, edited by Ray Droujkov.
Posted in Make
Calculus for Kids May 1, 2015 (Fridays)
This is the fourth meeting of a math circle inspired by calculus ideas. This week, we continue making an object in different ways. So the same Math Spark applies as last week (PDF).

Maria has returned from her trip to the Navajo Nation and brought something for the circle. It resembles one of the Math Words we’ve been talking about, integration. Let’s look at the design on top of the vase. What do you see?

This reminds us of Michele’s snail from last week.
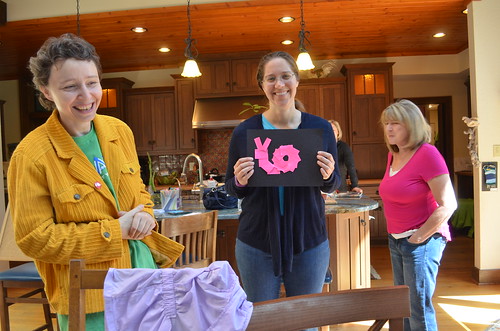
Maria is pointing out a symbol that goes around the circumference of the vase to Owen, and then to each child in turn: “What do you think this is?” Children think it’s a person sitting under the table, two letters L, and more – there are many different ways to read the same symbol! Maria learned from the trader that the original idea was two hands, symbolizing friendship.

What would be an example of 1D? The kids say a string of hair or a pen. Hannah answered by holding a pen up. Invite children to answer in many “languages” – words, gestures, and showing things.

We are brainstorming about dimensions, and seeing many different things again. “What do you see in the 3D LEGO sculpture?” A cow? A bison? A snail? Priyesh says “A mystery!”

Maria is illustrating dimensions using the pink yarn. Stretching the pink yarn horizontally, it is 3D.
 \
\
Now, squeezing the yarn flat, 2D. Imagine squeezing it into a dot to vanish into 0 dimensions.

Maria asked the children to take 9 LEGO blocks each (as many as there are people) and come together.

Hold the model of 0 dimensions (a LEGO block) and smile for the picture!

Each child integrated their “dots” into 1D lines. But then they wanted to add up all the lines together into a really tall tower (still a model of 1D though).


Would it stand by itself? “Abracadabra!” And then children wanted it to fall down, of course! It’s an irresistible desire when you build anything tall and narrow.

But if you attach the lines side by side, they model a 2D surface.

Here is the side view:

Uh-oh, 1 LEGO is missing! It turned out Julienne’s LEGO was missing, because she didn’t want to use a soiled LEGO piece on her tower (we got it replaced with a clean one). If we listen to kids and their small problems, everybody feels that they have a voice and power in the math circle, and it helps with mathematics.

Children brought up multiplication and its symbol, then addition, by using hand gestures. Maria added gestures for integration and infinity

Integration: lift up one hand to your side and make a curve and let the other hand curve down.

Infinity – hard to explain (hide thumbs in two side-by-side fists). Children said it does not look much like the infinity symbol, anyway.
It reminds us of a math dance cartoon:
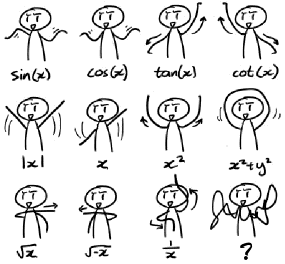
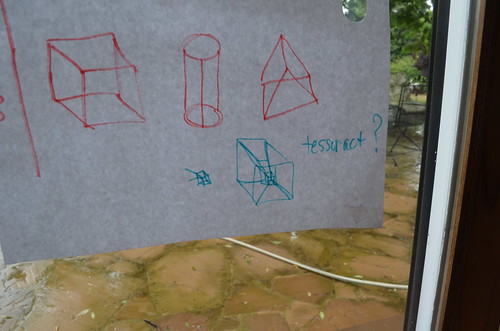
Parents had their own little math circle by the window while the children are working with Maria. They got all the way to the fourth dimension, looking up, discussing, and drawing tesseracts (4D cubes).
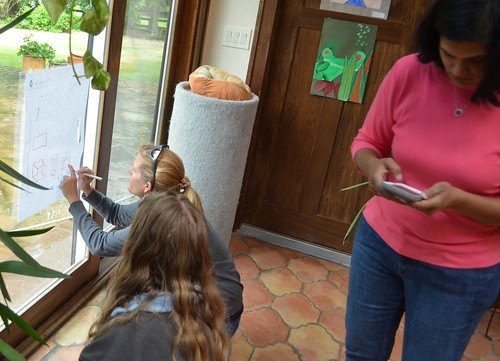
Meanwhile, children wanted to make the top layer pink, to show the 1D line across. It’s nice to see everyone’s working hands together – children in a small math circle, literally!


We kept that one green dot to stand for 0 dimensional point that can start a line… Then rebuilt the surface into a 3D cuboid.


Serrin is trying to make a circle out of towers to integrate in a different way, but the floor is too uneven. We move to the table and everybody works on their circle-integration ideas.


Maya’s circle – a line (1D) integrated out of points (0D).

Maya with her 3D LEGO creations: a diamond-shaped flower approximating a circle on the left, a pyramid approximating a cone, and a puppy.

Front and side views of the puppy:


Priyesh is working on a similar diamond approximation of a circle on the LEGO board.


Owen is about to make an abstract imaginative ghost from a 2D surface (coffee filter).

Julienne attached the LEGO blocks by sliding the bottom row half a block sideways.

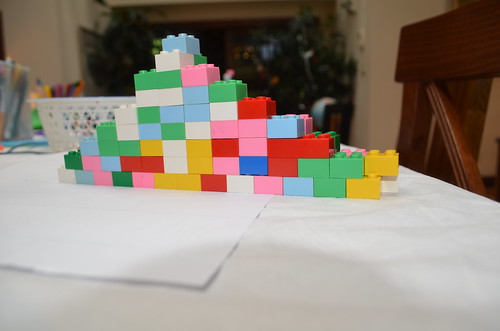
A paper model of our surface out of LEGO lines. This model is easier to roll into a cylinder than actual LEGOs.


What if we rolled Julienne’s LEGO staircase (approximating a triangle) around like that? What would we get? A pyramid? A cone? A cylinder?
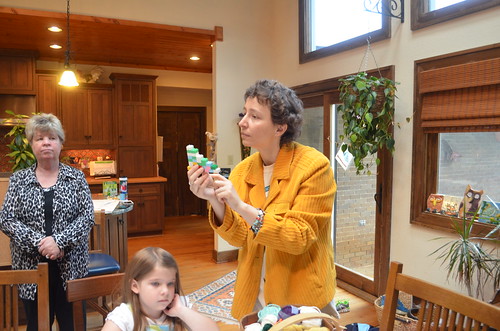
Maria cut a triangle out of orange craft foam to illustrate integration: “What shape would I get when I roll this triangle up?

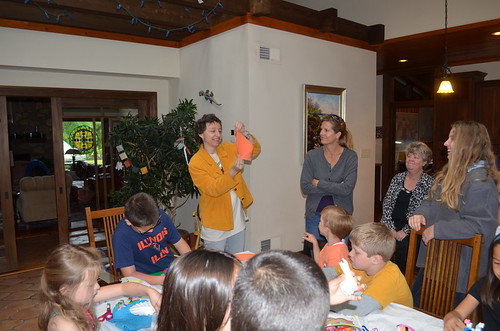
Moms are working on their triangles as well.

What should we call this shape?

When we try to define something that’s unfamiliar and new it can be both an exciting discovery and a frustrating experience!

Maria is about to show another example of integration, a cylinder made by rolling up a rectangle of craft foam.
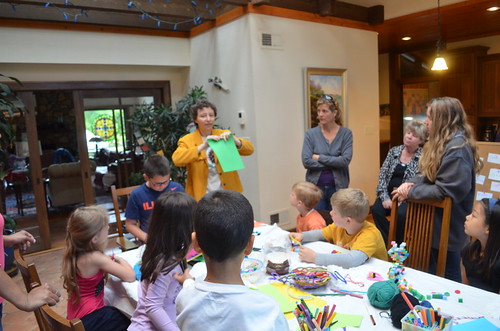
Jake building with LEGO blocks. He worked for a while figuring out the roof’s angle for his house. Slopes!



Jake decided to go back and work some more on his LEGO while the circle watched the video at the end. All the activities are voluntary, and sometimes children decide to work on their own projects instead. There are studies that show it does not prevent them from learning the content the rest of the group explores (they absorb it anyway). This ability to choose activities supports ownership and agency in mathematics.

Eashan cut out a triangle and drew straight lines across to show integration of a shape out of lines, then cut out the extras so it looked really neat.



Do you see beautiful mathematics in the chaos of papers and crafts? We do! From Eashan’s table:

Hannah’s volcanoes, integrated out of lava flow lines:

From Hannah’s table:


“Mess” by LoonarBaboon.com – believing is seeing in pretend-play and mathematics.
Priyesh and Serrin are trying to make a circle together, by using LEGO blocks on the board. Look at the chaotic busy working table in the background!

Learning is playing and playing is learning.

From Priyesh and Serrin’s table:

Smile, Owen! He made an imaginary plane (ship or cargo?) out of LEGO on the 2D green board. Check out symmetries.

Charlie is rolling up all three corners of a triangle – something grown-ups have not tried yet:

The circle is gathered around the kitchen table to watch “The Dot and the Line: A Romance in Lower Mathematics.”

Curious, amused, and giggly faces!

The views from afar and up close.



Photos by Erin Song, captions by Erin Song and Maria Droujkova, Math Spark by Kalid Azad, Shelley Nash, and Maria Droujkova, edited by Ray Droujkov.
Posted in Make
Moebius Noodles 2nd edition, and Calculus for Kids: Newsletter May 6, 2015
Subscribe and read archives
Pinterest | Twitter | YouTube | Facebook | Google+

In this newsletter:
- Moebius Noodles, 2nd edition: send us your questions and suggestions.
- Calculus for Kids – get inspired with photos and stories.
Publishing new and improved Moebius Noodles book
The first print run of Moebius Noodles, the book of adventures for young children and their grown-ups, is almost sold out. Good news is that we are about to publish the second edition.
Whether you’ve read the book or not, you can help us make its next version even better! Here’s how:
- Readers asked for more Questions and Answers. Send us your questions about Natural Math ways of learning, or about particular activities from Moebius Noodles. We may include answers in the book, or on the accompanying forum.
- We hear some readers feel inspired, but do not know how to start the activities with children. What helped you or would help you to begin your adventure?
- Have you noticed a typo or a style issue? Let us know!
Email your questions and suggestions to maria@naturalmath.com
Calculus for Kids project
We are running an experimental Calculus for Kids course online and two small local math circles in Cary, NC. We got tons of feedback on the activities, which will go into the next iteration of the course, the next round of math circles, and the book that will accompany them. Meanwhile, you can see lots of photos at the Natural Math site, and try some activities with your children.
Check out Calculus for Kids at https://naturalmath.com/tag/inspired-by-calculus/
See you online!
Dr. Maria Droujkova and the Natural Math crew
![]()
Posted in Newsletter






