Multiplication course and bananapples: Newsletter March 31
Subscribe to Moebius Noodles newsletters
Pinterest | Twitter | Facebook | Google+
I am Moby Snoodles, and this is my newsletter. Send me your questions, comments, and stories of math adventures at moby@moebiusnoodles.com

Math Spark: Bananapple!

When you start with a whole object and take it apart, your action can be a metaphor for differentiation. Find many different ways to slice the fruits and veggies you find: a perfect snack time activity! Then reassemble new crazy fruits out of slices. This can be a metaphor for integration.
For more detail, read the story from our local Math Circle.
Have a math spark from your family or group? Email us so we can share your adventures on the blog!
April-May open online course MULTIPLICATION: join 390+ participants!
I can’t wait to start the course. Hundreds of adventurous parents, math circle leaders, and teachers will invite their kids to share adventures inspired by algebra and calculus, art and storytelling, pretend-play and computer modeling.
Click to learn more and join the course.
Here is a picture from one of Week 2 activities about fractals, powers, and place values:

Why do people take the course? I read all the registration notes. I feel deeply touched every time I see the most frequent, simplest note: “For my child.” Here are some representative quotes:
- Glynn, USA: To continue to help me develop a math mind, so I can continue to help my kids do the same.
- Meg, Australia: We are homeschoolers. My 8 year old is very disengaged after spending two years in school. I am hoping to try something different to get him interested in exploring his world and I am hoping to learn more about following his lead.
- Serena, Australia: Homeschool with a nearly 5 yr and a 2 yr and a 9 month old. We love the Moebius Noodles book!
- Nike, England: As a volunteer teacher in an after school centre.Jessica, Canada: I am a K-3 Teacher and I am hoping to use this program to inspire my teaching.
- Lisa, USA: Homeschooling 12 year old with autism who has issues remembering his multiplication tables.
- Ellen, Belgium: With my grandchildren.
- Farrell, Canada: To help interest and educate my grandchildren in the joys of mathematics.
- Karen, USA: Fun with my grandkids.
- Jana, USA: I’m a classroom teacher in Adult Education/GED prep. I am particularly looking for materials to incorporate art in algebra! I have the PDF version of Moebius Noodles.
- Nancy, USA: I’m looking forward to using the math activities with advanced students in PreK-grade 2.
- Lezah, USA: I intend to see if I can implement any of the activities with my precocious 4 year old.
- Roberta, Canada: I have a 3 year old daughter and am looking for ways to teach her in a more natural and playful way than I was taught when I was a kid. She’s already learning so much through play!
- Gaelen, USA: Use it to get ideas for engineering things I do with little kids. Yadira, USA: I teach science and engineering classes to kids and thought this might be helpful.
- Kacey, USA: As a play therapist.
- Surabhi, Canada: I have a 3.5 year old boy I plan to unschool. I am looking for ways for him to explore his beautiful world and existence of math everywhere.
- B, USA: Just curious.
- Gilberto, Portugal: Private tutoring.
- Maureen, USA: I want to learn about math with my four-year-old son. He’s doing some multiplication at his Montessori preschool. I’d like to support at home but feel intimidated.
- Schuyler, Canada: I plan to use this to help me teach multiplication to my mixed-grade primary class.
- Katherine, USA: I have a math crazy 10 yo who loves math but still struggles memorizing her multiplication tables due to dyslexia. Thought I’d just try this out to see if it interests her and helps.
- Shannon, Canada: I plan to show it to my staff and fellow math teachers in our district during our monthly Math Inquiry project.
- Deb, USA: With my 10th grade who struggles with math (dyscalculia).
- Lian, Hong Kong: Supplement for my 3 y/o.
- Sara, USA: Help me get over my math anxiety so I can help my school-age kids.
- Manish, India: To teach math fun way to my children.
- Kate, USA: Planning to use this with my family and a math circle for 9-10 year olds.
News and reviews
Laura Grace Weldon did a detailed interview about Natural Math for GeekMom. One of my favorite questions: “On NaturalMath.com, you write about a community of people sharing naturally math-rich and meaningful activities for children from babyhood on. We’d love to hear about math circles and what you mean by math communities.”
The Italian journal D: la Repubblica published an interview about our work by Di Stefania Mendetti. Quote: “Alcune fra le opzioni più famose, ricorda Droujkova, sono Made in Italy, come il metodo montessoriano, sviluppato per individuare e correggere autonomamente gli errori e quello della Scuola di Reggio, che avvicina i bambini alla matematica attraverso progetti aperti.” English translation: “Some of the most popular alternative education systems, recalls Droujkova, come from Italy, such as the Montessori method, including self-correcting independent tasks, and that of the School of Reggio, which brings children to mathematics through open projects.”
A Turkish math and science site posted a translation of The Atlantic interview, by Merve Özçelik, with great illustrations such as origami snowflakes:

Sharing
You are welcome to share the contents of this newsletter online or in print.
Talk to you soon! Moby Snoodles, aka Dr. Maria Droujkova
Posted in Newsletter
Inspired by Calculus Math Circle – Week 2
Can you really teach calculus concepts to young children? Don’t they need to know algebra first, or at least arithmetic? We receive these questions a lot, and we do have our share of worries as we plan and lead “Inspired by Calculus” local math circles for 7-11 year olds. But let’s be brave together! Do try these activities at home by yourself (good), with your child (better), or invite some friends over (the best). If you do, please share your experience with us. As always, we welcome your questions and comments.
The first post in the series is here.
Second Meeting – Bodies of Revolution, Part 2
Our second meeting had to be rescheduled because of the weather. So kids had some extra time to play with the concept of integration (making big things out of many little things) using String Spin. Their new scavenger hunt task was to search for edible bodies and surfaces of revolution.
We started by revisiting integration. But we also worked on the concept of differentiation. Which brings up the question: “How can we show something this complex to young children?”
We find activities that serve as metaphors for the concepts, for example, differentiation as slicing an object into many parts.
First, kids tried to make some circles: “Remember that circles are made of triangles?” Children remembered this folding and cutting method from the last meeting, but it was still hard (most ended up with squares!) – so they were motivated to look for an alternative.
So out came salt dough. For the first few minutes the children just sat in a circle on the floor, divvying up the dough, smelling it, rolling and crumbling it, pounding it and mixing colors, and talking about it. This play time helps children to become familiar with properties of a new manipulative, before using them for mathematics.
Logistics won’t allow making salt dough with the kids during the math circle. But do try it at home! Making manipulatives together with your child is a powerful way to explore mathematics. For another good example, read this post about math of henna art by Rodi over at the Talking Stick Learning Center.
After a few minutes of free play, most kids ended up with some salt dough circles, made by squishing spheres or sculpting by hand. It was time to show them a more precise way of making a circle out of paper triangles (integrating).
At this point, children had done a lot of free play, both with physical objects (paper, salt dough, bodies of revolution they found/noticed around them) and software (String Spin). We noticed that their guesses (i.e. what curve would produce what surface in String Spin) were getting more precise – a good indicator that the kids were starting to discover patterns.
So the kids made their circles out of triangles until the salt dough got way too soft to handle. Then we returned to the unanswered question from our first meeting.
“What if we had a thick book with very many pages and we opened it so that the covers would touch. What shape would it make?”
This time, we actually had a cylinder made out of an old paperback book. And the question was more concrete: “What shape should we draw in String Spin so that when it rotates, we get this cylinder?” Interestingly, while all the kids recognized the object as a book, many came up with answers that looked nothing like a rectangle. Here are a few of their suggestions.
What was happening? It took us a few days to come up with the hypotheses that kids were trying to integrate 2-dimensional solids (book pages) out of 1-dimensional lines. We will test the idea next time. We modeled all children’s ideas using the software, but the solutions looked more like explosions or slices than book art. We’ll seek more precise software next time to do what we think kids want with integration. This was a very cool experience for the adults as it reinforced the following idea.
When choosing a math activity, it is not enough for the activity to be a metaphor for whatever advanced concept you want to teach to a child. It should also provide a way to help children communicate and share their math discoveries. And the best way to do so for young kids is not through words or formal mathematical notations, but by making things.
It was time to move to the metaphor for differentiation (in the grown-up eyes) – slicing (in children’s eyes). We asked participants to bring fruits and vegetables that were bodies of revolution.
Off we were to find yet another solution to our “how to make a circle” problem. All the kids figured out how to slice their fruits to get circles. So we asked them what would happen if we stacked the circles on top of each other? Would we get a cylinder, as some suggested? What if we stacked circles of different diameters? And what shape would we end up with if we kept stacking smaller and smaller circles?
And here came an interesting twist. Most kids insisted, even after modelling in String Spin, that stacking smaller circles would get us a pyramid. We are pretty sure that all the kids in the Circle are familiar with the words “pyramid” and “cone”. But we are also pretty sure that for now they do not distinguish between the two. One reason is that there has been, up to now, very little practical need for them to distinguish the two. The other reason is that a pyramid and a cone share a lot of essential mathematical qualities!
Which brings us to the question:
Shouldn’t kids know the difference between a pyramid and a cone, before working on calculus concepts?
From the calculus point of view, the difference between the two is insignificant. The process of differentiating and integrating and the volume formulas are the same for pyramids and cones. Overall, the sequence of mathematical education, what comes first and what comes after, is largely a matter of the established tradition. And the importance of knowing particular math facts or having certain math skills is relative to the area of the mathematics you want to learn.
Posted in Grow
Math mind hacks: Make mistakes on purpose

Turn making mistakes into a game. Make as many mistakes as you can in one problem. Can you make huge mistakes? Tiny, subtle mistakes? Clever mistakes and silly mistakes?
This game helps to prevent, or cure, math anxiety.
Here is Neil Gaiman’s speech on the subject.
Thank you for the poster idea, Julia Brodsky of the Art of Inquiry!
Posted in Grow
Multiplication course, bodies of revolution, global news: Newsletter March 17, 2014
Subscribe to Moebius Noodles newsletters
Pinterest | Twitter | Facebook | Google+
I am Moby Snoodles, and this is my newsletter. Send me your requests, questions and comments at moby@moebiusnoodles.com

Math Spark: Bodies of Revolution
Math Sparks are quick activities for grown-ups. If this one sparks your curiosity, share it with kids too!
Calculus studies how big things are made of little things. You can make some surfaces out of infinitely many copies of the same line, rotated in space. The best way to define solids and surfaces of revolution is by describing how to make them. If you’ve worked with a wood lathe, a pottery wheel, or an ice cream scoop, you are already familiar with the idea.
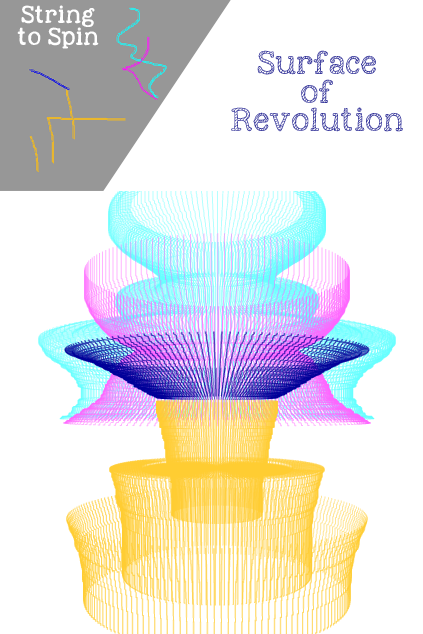
Here’s how mathematicians might give a constructive definition of a solid of revolution:
1. Draw a straight line.
2. Start at one end of the line and draw a squiggle that ends at the other. Now you have a shape.
3. Rotate this shape around the straight line
For a surface of revolution, you don’t even need to connect your squiggle to the line of rotation. Try creating your own surface of revolution with String Spin: http://www.zefrank.com/string_spinv2/
Any squiggle, even a random toddler’s doodle, becomes interesting once you spin it.
Moebius Noodles in the global news
My interview by Luba Vangelova for The Atlantic, 5-Year-Olds Can Learn Calculus, has generated discussions on blogs, forums, and news sites. I feel very thankful for the thoughtfu comments and deep questions. The themes that emerge from discussions make me cautiously optimistic. Many grown-ups believe that young math will finally give them a second chance at making sense of algebra and calculus. Others look for the balance between conceptual understanding and fluency at manipulating numbers. Even if 5-year-olds understand calculus, what would they use it for? Can we even call activities algebra or calculus if there are no formulas? Are young kids capable of abstraction?
At the same time quite a few people have come out saying that they are already playing advanced math games with toddlers or young kids – or that their parents did so with them! Our Natural Math community will be following up on these themes with an open event series, interviewing parents, teachers, researchers, and project leaders who work in related areas.
Want to join the discussion? Send me a story about math adventures of your kids, your students, or someone you know. It can be a cute thing your three-year-old said, or a game your mom made up for you when you were little, or an activity you adapted for your math circle. I am making a collection of happy young math stories!
Natural Math Multiplication: an online course in April

We invite parents, teachers, playgroup hosts, and math circle leaders to join us in April for an open online course about multiplication. Each week there will be five activities to help your kids learn multiplication by exploring patterns and structure. To get your course completion badge, do at least two activities every week. The course starts April 6 and runs for four weeks.
Each activity will have adaptations for toddlers (2-4), young kids (4-6), and older kids (7-12). If you want to remix activities for babies or teens, we will help!
Click to join the course.
Here is the preliminary syllabus.
Week 1: Introduction. What is multiplication? Hidden dangers and precursors of math difficulties. From open play to patterns: make your own math. 60 ways to stay creative in math. Our mathematical worries and dreams.
Week 2: Inspired by calculus. Tree fractals. Substitution fractals. Multiplication towers. Doubling and halving games. Zoom and powers of the Universe.
Week 3: Inspired by algebra. Factorization diagrams. Mirror books and snowflakes. Combination and chimeras. Spirolaterals and Waldorf stars: drafting by the numbers. MathLexicon.
Week 4: Times tables. Coloring the monster table. Scavenger hunt: multiplication models and intrinsic facts. Cuisenaire, Montessori, and other arrays. The hidden and exotic patterns. Healthy memorizing.
Sharing
You are welcome to share the contents of this newsletter online or in print.
Talk to you soon! Moby Snoodles, aka Dr. Maria Droujkova
Posted in Newsletter