4 projects from world-famous art exhibits your kids can do
There are math exhibitions held in many locations with many themes. The Bridges Conference and Joint Mathematics Meetings are two groups that seek to explore the many ways one might apply math to multiple disciplines, including art. The contributors to these conventions work in many disciplines ranging from mathematics and weaving to dancing and computer science and attract thousands of people annually. The Joint Mathematics Meeting of 2013 had over 6000 attendees!
These events are not only beneficial to adults. People of all ages can learn from art exhibitions. Seeing artwork inspires kids to start projects of their own and expand their artistic abilities.
From our “Do your little kids draw grids?” post
I went through galleries from the 2013 Bridges Conference and the 2013 Joint Mathematics Meetings and compiled some art pieces great to do with kids.
Jane Adler for the 2013 Bridges Conference
Jane Adler’s complicated looking tessellations on the quilt she designed for mathematician Marjorie Rice are as simple as drawing and coloring in triangular grids. Learning to work with grid shapes beyond squares can lead into more complex geometric thinking. In time, your kid or student will be working with diamonds, polygons, and more.
Horst Schaefer for Joint Mathematics Meetings
Using a combination of square and triangular grids, children can begin to draw mental connections with the way shapes relate to each other in a plane. Much like puzzle pieces fit together, so can polygons. Drawing these connections can inspire kids to look for connections in shapes throughout the world, first in a two-dimensional sense and then a three-dimensional one.
Marc Chamberland for Joint Mathematics Meetings
Marc Chamberland describes this exhibit as something even a child could do and he’s right. With markers or other coloring tools, paper, and scissors, kids can learn how squares are constructed from parallel and perpendicular lines and how even the pieces that don’t seem like they would fit together can form perfect squares.
Ton Oostveen for the Bridges Conference
Using a pencil and paper and the images found here, your child can learn how parallel and perpendicular lines can create the illusion of a three-dimensional wireframe. This teaches kids how three-dimensional objects present in the physical world are constructed.
Posted in Make
3D illusions, function machine props, tech for math ed online course: newsletter January 17, 2014
Got this from a friend? Subscribe!
I am Moby Snoodles, and this is my newsletter. Send me your requests, questions and comments at moby@moebiusnoodles.com
More about the multiplication project
For those who follow our multiplication adventures, here are more details. The next multiplication-centered event for parents will start with parent questions. Think of it as a citizen science project of the action research type. Action science is for a community solving a current problem using scientific tools. Together, participants collect data, analyze the data, implement the changes the analysis suggests, collect more data on how those changes worked, and so on – until great solutions to the problem emerge.
Picture from TheGriddle.net
We invite you to tell us what you and your children want about multiplication – and to figure out how to make these dreams come true! Depending on the level of community interest and support, the study may produce some or all of these results. As with all our projects, the results will be shared with the world, under a Creative Commons open license.
- THE MAP – The booklet “Multiplication: from worries to dreams” (PDF) with transcribed answers to selected questions from live webinars with parents and teachers.
- THE BIGGER MAP – We map out and briefly answer all the questions that did not make it into the webinars, and past questions from WOW! Multiplication course.
- THE INTERACTIVE MAP – We research and add links, resources, and full references to all answers, making everything is super-easy to find.
- NOW WITH TELEPORTS – We organize the resources into a web portal, Multiplication Planet, that is easy to use and invites further research and discussion.
Want in on this? Email me!
Blogs and networks
One of our most popular Pinterest boards is Eat Your Math. Check it out, and send us links or ideas for more tasty math activities!
Meet our newest blogger, pen name Marina Mersenne (check out the history), debuting with 3D Illusions with Easy Grids. Children often ask how they can create their own illusions. Your children can start with these art project ideas, and then experiment with other shapes and grids!
In the Math Mind Hacks series, check out Guesstimate. After I made the mini-poster, Sheryl Morris commented on our Facebook page that it would be nice to make a version for the Montessorians. The proof of concept comes from research of cognitive neuroscientists Park and Brannon, from Duke University.
We took part in the 70th monthly Math Teachers at Play blog carnival – a big collection of family math activities. For example, Mashka introduces her preschool/kindergarten math circle to thinking about systems in Tricky PreK Math, Lesson 8 – Year 2.
Take a huge loop of yarn (the size of half a room) and ask the kids to hold it at any place. Then tell them to close their eyes and that if they peek, the game will not work. Then, tell them that with their eyes closed, they have to make a square with the yarn. They are allowed to communicate with each other. Try it multiple times and see what happens! We only had a couple of minutes to play, but it came out very funny with everybody tangled. Almost all of them were cheating (their eyes were open) and they still couldn’t make a square (or even get untangled).
Questions and answers
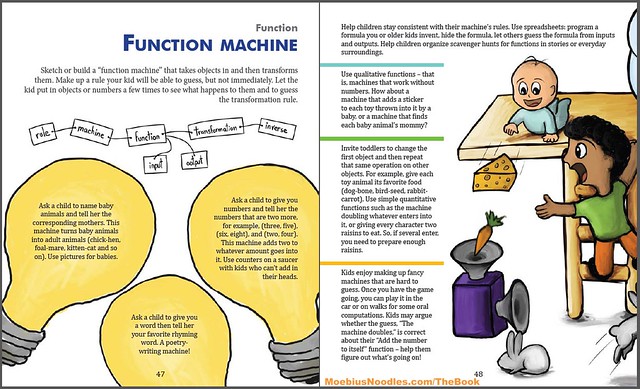
We have a playschool group with kiddos 0-5, with moms, and were thinking about acting out function machines with one mom in a box and we hand her input (toys, numbers) and she give us output (altered, increased, decreased). Has anyone done something similar and can you recommend what props are good to have on hand to make this fun?
Share your prop ideas at the forum! Meanwhile, here are some suggestions:
- Boxes with both ends open, or tubes (you can just tape paper into a cylinder) are more fun to use than a flat Function Machine drawn on paper.
- Heads up: young kids want a new function machine for every new function they make up, or you make up. For older kids, the same diagram or just the letter F can stand for different functions, and the same prop can represent different machines. Little kids won’t like that, at least at the beginning. Later you can introduce a magic wand or a wrench that kids can use to change and modify your old function machine they already explored into something new and exciting. You can use a pencil to pretend-play that tool, or bring a strange-looking shop tool or utensil for your prop.
- Food you can slice, such as apples, works great for machines that cut things into halves or other parts. Raisins are nice if the machine houses something like Purple-Tailed Raisin Eater, who always swallows two of the raisins that enter and no more.
- Playdough or soft modeling clay can create interesting machines. You can slice it, stretch it, squish it for a variety of 3D transformations.
- Small stickers or cards plus large markers can be props for coloring machines that turn everything that enters green, red, or polka-dotted.
In general, think of machine rules ahead of time, and how you would pretend-play them!
We have several activities in the Moebius Noodles book, which also has some suggestions for prompts.
An open graduate level course for teachers and parents: Technology for Math Ed
I am leading a semester-long online course at Arcadia University. As usual for the courses I design, all course assignments will happen in live online communities, and people who are not at Arcadia are welcome to participate. You can view the syllabus and jump right in with the first assignments! Here is the plan, by weeks:
- Introduction, setup, review of your goals and needs
- Tech we use for the course
- Meeting one another. Who are you? What do you want in math ed?
- Topic brainstorm: what will your personal week be all about: Take 1
- Mathematical modeling and its pedagogical uses
- Models of math vs. modeling (of other entities) with math
- Make and share, or consume? Roles of students in modeling
- Models and multiple representations
- Example of modeling software: GeoGebra
- Crowd-learning: networks and communities
- The cultures of sharing: Pinterest, Tumblr, Flickr…
- Mathematical problem-solving with computers and social tools
- Examples of social phenomena: Scratch and Project Euler
- Topic brainstorm, Take 2
- Our Wikipedia article, Take 1
- Hands-on tech
- Manipulatives
- The maker movement
- Comparing and contrasting virtual and physical worlds
- Examples of physical and virtual tech
- Crystallized and fluid intelligences
- Solvers, calculators, graphers, and sending humans to do robotic jobs
- Problem-solving, problem-posing, conjecturing, proving: creating and evaluating in math
- Computer superpowers and what you can’t do without
- Examples of computer-based and computer-delivered math: Wolfram|Alpha and Khan Academy
- Humanistic mathematics
- Math-rich digital arts
- Computational origami, hyperbolic crocheting, fractal cookies: 21st century crafting
- Interpretive dance and underwater basketweaving: issues of intellectual honesty
- Examples: Bridges conference and Gathering for Gardner
- Accessibility and diversity
- Learning styles
- Tech for boys and girls
- Disabilities and learning tools in mathematics
- Advanced math without prerequisites (young kids, ESL, troubled learners)
- Example: ethnomathematics community
- Our Wikipedia article, Take 2
8. Maria’s topic of interest: algebra and calculus for very young kids
9-14 Your topics of interest (to be determined in earlier weeks)
15. Summaries and farewells
Sharing
You are welcome to share the contents of this newsletter online or in print.
Talk to you in two weeks! Moby Snoodles, aka Dr. Maria Droujkova
Posted in Newsletter
3D Illusions with Easy Grids
From Escher and the Droste Effect
In many ways, graphic art is the visual interpretation of math. Grids are a great example of this.
From the Math in Your Feet blog
Grids are found everywhere in art. In fact, your kid or student may be drawing grids already, like the ones we described in “Do your kids draw grids?” post. If you want to contribute pictures of your kid’s grids, email us at moby@moebiusnoodles.com
Drawing grids involves building two-dimensional shapes using a row and column structure. Visually exploring these two dimensions helps develop spatial and algebraic reasoning.
Grids are a good starting point for understanding two-dimensional shapes, and the same spacial reasoning can be used to give the illusion of three dimensions. By warping grids in parallel with a figure, a person can create the optical illusion of volume where there is none.
From Color, Craft, Create blog
Using nothing but paper, a circular object such as a cup, and a pencil or two, you can introduce your kid to the wild, wild world of three-dimensional volume. The project instructions found here show how you can create the illusion of a sphere by adding parallel lines to the two-dimensional shape.
Using a ruler, a pencil, coloring supplies, and these simple instructions, your kid can easily create an impressive work of art using parallel lines and complementary colors that accentuate the 3D illusion.
A common tradition in kid’s art is drawing hands, so kids will be comfortable tracing their hands in order to do this colorful 3D project.
Because of the use of grids, even kids who can’t draw well will be able to create these vibrant, engaging pictures.
Drawing colorful pictures is a great project that will get your kid excited about even more adventures with math art.
Have fun!
Posted in Make
Math mind hacks: Guesstimate
Photo from Technology Rich Inquiry Based Research.
Estimation is a skill everyone uses. You estimate how much cheese is enough for your party, or how long it takes you to drive to your friend’s house. But some people estimate much better than others. Why so? Cognitive neuroscientists Park and Brannon have two pieces of good news you can use.
First, their study confirms that you can train your Approximate Number System (ANS) to improve your guessing and estimation skills. This is not surprising: most math-related skills are trainable. Here is the more exciting, brand-new finding: guesstimating improves your ability to do symbolic addition and subtraction.
From the abstract: This finding strongly supports the hypothesis that complex math skills are fundamentally linked to rudimentary preverbal quantitative abilities and provides the first direct evidence that the ANS and symbolic math may be causally related.
Update: Sheryl Morris commented on Facebook that a Montessori bead version of this mini-poster would work well. Here it is.
Photo from Montessori Album.
Posted in Grow
