Inspired by Calculus: Tuesday math circle, Week 3
Please shoot me a quick email if kids do or say anything related to functions, infinity, and other such topics, at home. It really helps to plan for the next time, and it’s always interesting!
Thank you for taking notes, Kristin, Bill, and Guy! I use full notes for write-ups. And thank you everyone who helped with photos. Here is our full photo set.

Share something you love. Ben brought a set of building magnets. Kids found geometry and infinity in it.

Kim built a hexagon out of small counters, and kids found infinity in it.


Maxime made the diagram of her family tree (it’s a binary tree, like we made last time) – and said that family, and people, can go to infinity. Kids grew their math eyes: now they see infinity everywhere!
What did we do last time? This is an activity about math terms, and about math connections. As kids recall what we did, I ask them if they want to add math words to our board. As a participant, I get my turn adding words, too. You probably noticed that we take turns a lot. Grown-ups often share the knowledge of past generations (existing math) when it’s their turn. Children often make something up, or at least make new connections. Even on mundane tasks such as naming terms, we can expect the unexpected from children’s divergent thinking and creativity. Tradition+creation=progress. At home: try to find some of our math words in the world around you.

Use counters to guess function machines. Three counters go in, and five come out. Four go in and five come out. Can you guess the rule? Children created their own machines and guessed one another’s rules. The goal is to connect the idea of function to calculus ideas, such as limits. At home: play the function machine game without counters, just giving inputs and outputs as numbers. Or use objects of your choice to model function machines.
Negative zero? This was a short spontaneous discussion from something Stephen said. None of the kids had ideas on what a negative zero could mean. That’s one reason I invite parents to participate. Alicia suggested approaching zero from the left (on the number line). Children liked the idea! It will come handy later, when we work more with limits. Kids often say weird things that you can unfold into interesting, deep math.
Accidentally, exponents! “What I noticed today: when kids worked with LEGOs, they made these exponential functions…” – Alicia told me after the Circle was over. This observation, and our conversation about it, started a train of thought I’d like to share.

When we worked with the counters and drawings of “function machines,” all kids designed linear functions. Their machines multiplied or divided by a number; or their machines added or subtracted a number. This is a consequence of the existing math curricula and math materials. Even if kids have been exposed to non-linearity, as I am sure all these kids have been, the bulk of their number fluency is linear.
The task was to build LEGO towers out of our function machines: bar graphs, though we did not use the term. The first tower was the output for the input of 0, the next for the input of 1, the next for the input of 2, and so on. But this is not what most kids actually did! When working with LEGO, most children used their formulas recursively: that is, they applied the rule to the previous output.
There are two strokes of genius in this change the kids made. First, iterations greatly simplify the structure of input-output functions. Instead of keeping accounts of two series of numbers (inputs and outputs), and their connections (this input for that output)… Children only need to keep track of one series that builds on itself. Here are side-by-side comparisons for Kaiya’s rule (+5) and for Jason’s rule (*3), with the towers they made.

Elena came up with a hybrid way to represent patterns. “One goes in, two comes out… Two goes in, four comes out…” She iterated x2, but she represented both inputs and outputs in one line: 1, 2, 2, 4… The horses were doubling, too.

If you count the LEGO bricks in the pictures, you will see some of the patterns change mid-way. Are these mistakes? Why didn’t we fix them? Most of the time, you see breaks and mistakes in patterns when kids get a bright idea. Kids can either chase a new idea, or keep the old pattern going, but there is not enough both. Do not pay too much attention to mistakes – instead, ask kids what they are thinking. You can chase the new idea together, and fix the pattern later. All the kids in this group pointed out breaks in their patterns. Don’t be afraid children will forever miss a mistake. Losing new ideas is more dangerous.
Chris wanted only whole numbers to come out of his function machine that divided by three. So he skipped the inputs of zero, one, two… four, five… seven, eight… and so on. Kids often have very particular wishes about domains of their functions, either because they prefer computations to be nice, or for aesthetic reasons.

Malcolm used volume rather than height to represent his function, because there weren’t enough bricks, he said. We may follow up on that idea and do more with volumes next time. Yay for creative solutions!

“Make things as simple as possible, but not simpler” – Einstein explains about a core principle of math and science. To make things simple, you often kick the abstraction up a notch. Kaiya: “It starts with two [this is a choice; she could start with any number – Maria], and goes to [pauses to check again] seven, which is five more. And then it goes to five more than that… whatever it is. And five more than that, again.” Note the phrase – whatever it is – a child’s way to describe variables. This is the language of abstraction, of algebra or calculus. We don’t care as much about particular numbers, as we do about the patterns. Because we are making things as simple as possible, we want algebraic patterns! A pattern is simpler than listing all the numbers in it. At home: find ways algebra helps us simplify pesky calculations. Celebrate simplicity.
You might have noticed that using the same rule as input-output (with inputs of 0, 1, 2, 3…) or recursively gives different results. This is the second stroke of genius. Kids accidentally produced nonlinear, exponential systems when they iterated multiplication. We now had examples in the group to make some nice comparisons between linear and nonlinear functions – the goal of the whole day.
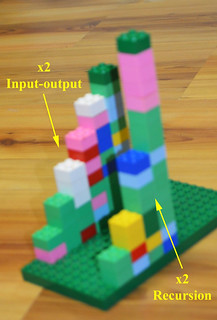
Why make things as simple as possible? Because you can deal with more complex things!
“But it is always straight if you only use two towers” – Stephen explains a property of a function, any function. We used straightedges to check if each set of towers was growing “straight like a slide” or “curvy like a roller coaster.” Children enjoyed making predictions, and then checking. If you only use two of the LEGO towers – whatever the overall pattern of towers! – you can always make a straight line between them. Drawing a straight line between two points on a curve is exactly what you do to find tangents, and to perform numeric integration, and in myriads of other calculus tasks. We will continue with this line of thought next time.

Video of the day –Sierpinski Dream

Posted in Grow
Inspired by calculus, grid art, street math: Newsletter October 15
Got this from a friend? Subscribe!
I am Moby Snoodles, and this is my newsletter. Send me your requests, questions and comments at moby@moebiusnoodles.com

Blogs and networks
Two weeks ago, we started several local Math Circles in our new Inspired by Calculus series for kids ages 4 to 10. Read detailed reports at the Moebius Noodles blog, see young math faces, and try it at home.


Our Facebook fans, in response to Robin Angotti’s funky iPad project, discuss childhood math objects that become treasured and special. Nancy Winans writes: “I remember a round plastic purse I had as a child around age 6 – a picture of a girl with the same purse with the same picture of her and it went on and on just like this for several repetitions that I could see. I remember looking at that image many many times and pondering it.” Yelena McManaman adds: “I had a huge collection of buttons and ribbons and I remember sorting and arranging the buttons lots of different ways and building with them, mosaic-style.” Do you remember any math objects from when you were little?

We hosted the Math Teachers at Play blog carnival #67! My personal favorites are modeling tasks like Minecraft optimization, and deep math ideas for young kids, like graph theory with dolls.

What do you see when you look at drawings by little kids? For example, grown-up artists noticed that many young artists draw big heads with legs. The more people talked about these shapes, the more meaning they found. By now developmental psychologists have come up with big theories around these simple observations: “Tadpole drawings appear during the pre-schematic stage…”

Grown-up mathematicians noticed that many young mathematicians draw grids. Round grids, square grids, grids within grids!
Have you kids made any grids? Please send us pictures and stories! See more example in our blog post, Do your little kids draw grids? with masterpieces from Michael Kelly’s son Francis. Thank you to all who sent us grid examples since we posted this!

By request from our newsletter reader Anna Belaschenko, we compiled math projects for the outdoors: 6 BIG adventures in street math.
Events
Yelena McManaman and I held a family math event at SAS. One of the interesting parts of the discussion was brainstorming different Math Circles, from a math tea party for girls to a grandmothers/grandchildren club. It’s always great to try new formats.
Write if you want to have us for an adventurous math event at your place.
Sharing
You are welcome to share the contents of this newsletter online or in print. You can also remix and tweak anything as you wish, as long as you share your creations on the same terms. Please credit MoebiusNoodles.com
More formally, we distribute all Moebius Noodles content under the Creative Commons Attribution-NonCommercial-ShareAlike license: CC BY-NC-SA
Talk to you again on October 30th!
Moby Snoodles, aka Dr. Maria Droujkova
Posted in Newsletter
Inspired by Calculus: Friday math circle, Week 1
These are notes from a Math Circle for children ages 4-5 and their parents. The goal of the first meeting on October 11 was to introduce cycles, iterations, and infinity. We had about a dozen quick activities, half of them from the book Moebius Noodles: Adventurous Math for the Playground Crowd, and the rest newly collected for this Circle.
Here is the set of photos from the Math Circle. I love watching children’s math faces. When children concentrate, or find a new idea delicious, or debate a paradox, their faces are mirrors of their mathematics. At home: pay attention to your children’s math faces. Capture them on camera.
Draw what you like! Say what you like! I try to start every Math Circle with some sort of sharing of what kids like. Sometimes we find math in these lovable things. Value affirmations before challenging journeys help the flow and the overall well-being. Note that some kids were drawing with two hands, as I suggested. You need to tape paper to the table first. This helps with creativity and math thinking, in somewhat indirect ways. I often do this doodle game with kids before doing other math activities.
Introductions. I bonk people on the head (lightly) and they say their name. I started to do it a while ago, because many kids freeze if you point at them and ask them to talk. Nobody freezes if you bonk on the head. This is a very simple example of a very deep math ed principle: use the body to do your math.
Spontaneous activity: Grid pictures. Pictures that look like grids, railroad tracks, wheels with spikes, or concentric circles appear in kid art sometime around the age (or instead of) “tadpole drawings” – and persist from then on. Some kids get a bit obsessed drawing grids! You can read more around the grid pictures at the Moebius Noodles blog. Mathematically, grid pictures are important for multiplication and algebraic laws, such as “distribution” of a line across all other lines. At home: notice if your kids draw grids.
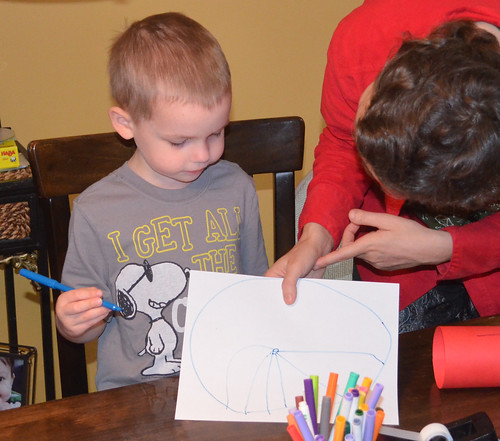
Function machines. We only looked at one function: it added a counter to whatever number of counters we already had. Kids wanted me to “work the machine” again and again and again; Dylan helped to make the machine work. I asked children to draw as many counters as will come out next time, and we checked by placing them over the drawings. Kids need to answer questions by doing, rather than only words. At home: make up your own function machines.

Cut a piece of paper in half… Again and again and again! This is an example of (potentially infinite) iterations. It’s very popular with kids of all ages, even kids who don’t usually like to cut with scissors. Children try to make their pieces as small as possible, or experiment with shapes they can make.

Grown-ups often arrange the pieces nicely, and help children see the patterns. Young ones don’t initiate this patterning, but appreciate it; that’s why mixed-age groups rock. At home: slice or fold more things – again and again and again, into infinitesimal pieces!

Going around in circles. Some kids sat on the table, and Mason and I walked around. Together, we said the name of each kid we met: “Asiya, Lucas, Dylan, Traver, Asiya, Lucas, Dylan, Traver, Asiya, Lucas, Dylan, Traver…” Then we tried with the eyes closed. It’s hard for some kids. Then we tried backwards! It was hard, but Asiya could do it, even with her eyes closed. Doing math with the whole body… Doing math with names… These two simple technique help young kids relate to math! At home: play memory games with repeating patterns your kids make up. Find some situations when repeating patterns happen, such as taking turns in a small group, or frieze designs.
Ring around the rosie… with your eyes closed. Circle games are traditional in many places in the world. They often symbolize cycles of seasons. At home: invite kids to do math with their eyes closed. This simple trick, often used in Montessori activities, helps kids to concentrate, to get in touch with their bodies, and to focus their attention and memory on key math attributes of the situation. It also helps kids to relax and to laugh.

Where do we see things that happen again and again? Kids sort of froze at that question, and I sent them to ask parents. This is something I will do – again and again and again! – in Math Circles wherewhere kids don’t know what to do next, or when we need to deepen our activities. Kids understand their own parents really well, and can explain to the group what parents say. It just felt so good, and it was funny to see families whispering together, and kids were so eager to share the ideas afterwards.

Breakfasts, bedtimes, seasons, sunrises – a lot of iterations revolved around the Sun, so to speak. Notice that kids recognize and appreciate much deeper ideas than they can produce themselves. That’s why we read (more complex books) to kids even after they learn to read, and listen to (more complex) music than we can play, and expose them to more complex math than they would be able to produce! Iterations in your body. I helped kids to observe blinking, breathing, heartbeats – some body functions that happen again and again and again. At home: find more things that happen again and again.
Powers of the universe movie. I usually finish Math Circles with a video. Kids can watch videos even when they are tired; they see complex math in beautiful, accessible ways; and they often share the videos with friends and family. This day, we watched the classic “Powers of Ten.” Mathematically, it’s all about zoom. At home: play with zoom in computers, cameras, or your imagination!
Posted in Grow
Inspired by Calculus: Thursday math circle, Week 2
This week, we tackled the idea that infinitely many things don’t have to add up to infinitely large space. The idea is deep, but young children don’t have the mental resistance to it yet. But they do feel the sense of wonder! In general, you want to introduce ideas early enough that they don’t cause too big a conflict with the kid’s previous thoughts on the Universe…

We have detailed notes about kids – thanks a lot, Lynna and Sally! Here is the link to all the notes. It will help us a lot when we write up these materials for other Circle leaders. Thank you for taking photos, Svetlana! Here is the link to all the photos of this Math Circle.

I am very happy with every participant’s depth of thinking, creativity, and persistence with hard concepts. You probably won’t see me praising kids (or persons in general), because in the long run, it interferes with the flow and the motivation. But I try to praise interesting ideas. And we do see lots and lots of interesting ideas.

What interesting things happened this week? Where is math in there? This combination of a scavenger hunt and a show-and-tell brings home the message that interesting math is everywhere. For example, it’s in the programming of a robotic spider Luca installed at home, and shared by drawing. At home: seek math everywhere!

Does Hotel Infinity take up infinite space? The math motivation is to approach limits. At home: find examples of “infinity pieces” that do, or do not, take up infinite space.

When I did this activity on Tuesday, I asked kids this question in the abstract. This time around, I asked them to draw the model of Hotel Infinity on paper, and see if they can make ALL the rooms fit. We had a curious problem that I (half-jokingly) attribute to gender: all the little boys wanted to expand the space of their hotels, even by attaching extra sheets of paper – rather than “go into” smaller and smaller pieces! But we got a good discussion out of comparing these two scenarios. When do you need more and more paper? Can you fit infinitely many aliens into a finite hotel, if the aliens get smaller and smaller just like rooms? Roman came up with a very meditative doodle method, reminding me of Vi Hart videos: lots and lots and lots of small doors…



In general, kids and the parents doing the Circle had divergent, creative fun with hotel designs – spirals, fractals, going into 3d… We need to expand this design activity some more.
Zombie binary tree. This was our move-around activity for the day. We built a binary tree out of ourselves, pretending to be zombies, because the hands were stretched out front. By this time, kids really wanted to run around, so the activity was a bit hard to do. We need more move-around activities for the younger groups! Binary tree is a type of a fractal, and a nice photo-op for large groups. At home: show math ideas with your whole body(s) for great photos and videos!
Make a square out of paper. It’s a Maker task, and as such, requires design decisions: every kid made a square in a slightly different way. Gray even declared that his full rectangle of paper is a square – I suggested it can definitely happen, on the Hat Planet, that we call that shape “square.” At home: invite kids to figure out their own ways to make something, from light saber-shaped pancakes to furniture-and-sheets forts, and name it in their own ways.

Half of a half of a half. Kids get a lot of joy out of cutting paper smaller and smaller and smaller… So maybe my hypothesis about the need for space expansion is all wrong! Hayden even asked for tweezers to handle the tiny pieces of paper. This is another take on limits: a particular example of an infinite series of shapes that takes up finite space. We used our squares. If you cut the square in half, then add half of (the other) half, add half of a half of a half, and so on… What number do you get as your sum? I am still to ask this question coherently. What I need is a way for kids to come up with a lot of different examples of finite limits. The example where the answer is one is not a good start (even if it’s a good activity): you don’t ever want to start new math concepts from numbers such as 0 or 1.

Even drawing that process on paper (Hayden said it reminded him of the Nautilus) did not help to understand that question. Still, we had a good discussion amid the vagueness! Jeremy thought the sum may be Pi, and Pi definitely has to do with infinite series, being irrational… At home: cut things again and again and again, put them back together, discuss infinity.
Infinity elephants movie. This is Vi Hart’s artistic take on limits. At home: watch this again, discuss it, watch other Vi Hart’s movies. http://www.youtube.com/watch?v=DK5Z709J2eo
Posted in Grow




