Math Storytelling Day: Featuring Carol and the CHS Teen Algebra Club
Today is The Day! March 25th, the Math Storytelling Day is here. And we are sharing with you math stories (in verse and in drawings) sent by Carol and the students at the CHS Teen Algebra Club. Telling stories helps us to understand our students, to make mathematics beautiful, and to reach out to others in the Natural Math community. 
Do you have a story to share? Perhaps it just happened today or this post reminded you of something that happened a long time ago. It is not too late to
- Make the word MATH out of objects you and your kids love and use, and take a photo, or…
- Tell us the cute thing your kid did, or math adventures from your math circle, or what happened to you as a child, or…
- Just finish the phrase: “For me, math is…”
Send your pictures and/or stories to moby@moebiusnoodles.com and tell us how to credit your picture: your name(s) and location. For every 20 stories we get by the end of the day today, we will randomly select one. Its author will receive our official limited edition MATH IS WHAT YOU MAKE OF IT! t-shirt.
Carol, the Headmaster of Heroic University, where they celebrate and develop heroes in all disciplines and all walks of life, submitted this limerick:
In my quest for a worthy math hero,
I’m finding who created zero.
Babylon and Mayans,
And then Indians….
Why’s Brahmagupta less known than De Niro?
The students participating in the CHS Teen Algebra Club decided to make the word “MATH” using a grid. So inspiring! Happy Math Storytelling Day to all!
Posted in Make
Boomerangs for peace, a cognitive experiment to try, math stories: newsletter September 16, 2014
Subscribe and read archives
Pinterest | Twitter | Facebook | Google+
Hi, I am Moby from the Moebius Noodles project, bringing you the news about Natural Math. Send me your questions, comments, and stories of math adventures at moby@moebiusnoodles.com
In this newsletter:
- Easy to make boomerang – try it at home to join a global project
- A cognitive science experiment with your kids, or grown-ups
- But you can split any number if you have a knife! Math Storytelling Day is September 25th – share your story!
1001 circles and leaders: Boomerangs for world peace
Professor Yutaka Nishiyama（西山豊) seeks mathematics in everyday life and in cultural traditions. How does an egg roll down a slope? Why do French, Japanese, and Indian people count on their fingers in such different ways? Why do boomerangs fly? Yutaka finds out through research so playful you can follow it with your kids. I love how his accessible explorations mix childlike curiosity, the DIY spirit of making, the scientific experiments – and global sharing. In 1999-2007, The International Boomerang Project for world peace translated instructions for making simple paper boomerangs into 70 languages, making it accessible to 99.99% of the world’s population.
Make your own paper boomerangs with easy instructions from Yutaka, or leave a comment for him on our blog: https://naturalmath.com/2014/09/1001-leaders-make-and-fly-boomerangs-with-yutaka-nishiyama/
Babies, young kids, and even grown-ups can use their Approximate Number System for algebra
This week, we interviewed Dr. Melissa Kibbe about her cognitive psychology research with young children. Dr. Kibbe answered some questions for our blog, and then came to our virtual studio for a live event in the Math Future series. She writes:
Infants, children, adults, and some animals all have a built-in approximate “number sense”. We use this number sense whenever we want to, say, compare which of two jars has the most jelly beans or when we want to estimate how many people are in a crowd. We asked whether children could harness their approximate number sense to solve the kinds of problems that are usually not introduced much later in formal schooling, and with which children often struggle. That is, we thought that presenting problems in a more intuitive way may help kids “solve for x”.
You can try this experiment at home. In our studies, we show children problems with unknown addends (like 5+x=17), but we present them in an intuitive way, embedded in a story. Children saw a stuffed animal character, Gator, who had a “magic” cup that will add more objects to a pile of objects, such as buttons or pennies. Children get to see the piles before the cup added its quantity, and after. We showed children the “magic” cup working on different piles, but always adding the same amount every time. Then, we pretended to mix up some cups, and showed children the quantities inside two different “magic” cups, only one of which matched the quantity that Gator’s cup had added; children were asked to choose which cup belonged to Gator. Children were quite good at choosing which quantity was in the animal’s cup, showing that they had an intuitive sense of the quantity of the unknown addend. Parents can try these kinds of activities with children at home, using items that are found around the house and a favorite toy.
Math Storytelling Day September 25: some stories!
Celebrate Math Storytelling Day by sharing your math stories, big or small. One in every 20 people who submits a story before September 25th gets a Math Maker t-shirt.
In this story, Irina caught her daughter making her own mathematics – namely, her own definition of a key idea:
My six year old daughter Alexandra loves math.
She also loves apples and sweets, and occasionally is willing to share food with her brother. In May we have discussed how we can fairly split up pizza, pies and apples between 2, 3, 4 and more persons. What if one person donates his share for others? Alexandra quickly grasped the concept of fractions and started playing with them in her head.
One month ago we were reading and doing exercises from a book called, What Should I Know Before I Start School? One chapter tried to introduce types of numbers: those in blue boxes (1, 3, 5, …) were odd and those in orange boxes (2, 4 ,6, …) were even. “If you have an even number of items, you can split them evenly in two groups” – the book said to my bitter disappointment.
After some thinking, my daughter came up with a new definition: “Even numbers of apples and sweets can be easily and fairly between me and my brother without cutting. For odd numbers of apples, we would need a knife.”
We are posting stories and pictures to our blog. Send us your stories, big and small!
Sharing
You are welcome to share this newsletter online or in print.
Talk to you soon! Moby Snoodles, aka Dr. Maria Droujkova
Posted in Newsletter
Math Storytelling Day: featuring Sheryl, Irina, Anna, and Jevanyn
Today, we share with you math stories sent by Sheryl, Irina, Anna, and Jevanyn. Telling stories helps us to understand our students, to make mathematics beautiful, and to reach out to others in the Natural Math community.
To celebrate Math Storytelling Day on September 25, we invite you to share your stories! Here’s how:
- Make the word MATH out of objects you and your kids love and use, and take a photo, or…
- Tell us the cute thing your kid did, or math adventures from your math circle, or what happened to you as a child, or…
- Just finish the phrase: “For me, math is…”
Send your pictures and/or stories to moby@moebiusnoodles.com and tell us how to credit your picture: your name(s) and location. For 10 more days (till the 25th), every time we get 20 submissions sent to us, we will randomly select one, and its author will receive our official limited edition MATH IS WHAT YOU MAKE OF IT! t-shirt.
Sheryl Morris arranged the word MATH out of her favorite objects-to-think-with:
Irina Malkin Ondik caught her daughter making her own mathematics – namely, her own definition of a key idea:
My six year old daughter Alexandra loves math.
She also loves apples and sweets, and occasionally is willing to share food with her brother. In May we have discussed how we can fairly split up pizza, pies and apples between 2, 3, 4 and more persons. What if one person donates his share for others? Alexandra quickly grasped the concept of fractions and started playing with them in her head.
One month ago we were reading and doing exercises from a book called, What Should I Know Before I Start School? One chapter tried to introduce types of numbers: those in blue boxes (1, 3, 5, …) were odd and those in orange boxes (2, 4 ,6, …) were even. “If you have an even number of items, you can split them evenly in two groups” – the book said to my bitter disappointment.
After some thinking, my daughter came up with a new definition: “Even numbers of apples and sweets can be easily and fairly between me and my brother without cutting. For odd numbers of apples, we would need a knife.”
Several pages (and days) later, she was asked to fill in the blank in this number sequence:
1, 3, 5, __, 9, 11
When I asked her how she has solved the problem, Alexandra replied: “It was easy. Those were odd numbers.”
Anna Kuchment invited her daughter to check out our Math Mind Hack mini-poster. Anna had a question: “How do you discuss this with your child? Do you ask how do you find the area of this square, or what is five times five?” – and her daughter helped. You get such surprise gifts as an attentive parent open to child’s ideas! Anna writes:
I went ahead and showed this to my 8 year old daughter, and she had so much fun with it. I didn’t notice the five fives on the starfish, but she picked them out right away. I think the lesson here is that children are far more fearless when it comes to math than most non-STEM parents! Lesson learned.
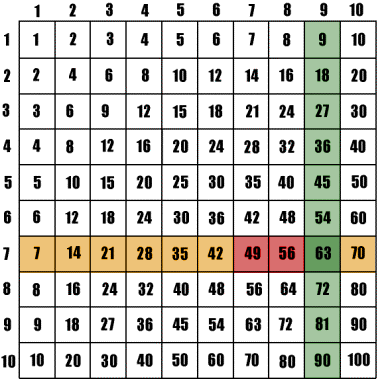
Jevanyn shares a story of turning mundane homework exercises into a mini-investigation of patterns:
Like many 3rd graders, my son is trudging his way through the times tables, learning multiplication and division the old-fashioned way. Tonight’s homework included:
56 / 7 = ?
and
49 / 7 = ?
Aaaand apparently they haven’t actually reached the sevens part of the times tables yet, or they have and he’s mentally blocking it because ermagrd homework.
So the goal here is to help him find the answer without telling him outright. I start (probably not helpfully) by asking him how far apart 56 and 49 are. So he counts up from 49 to 56, and gets 7. We’re getting somewhere.
Now I say, “So if 56 is some number of sevens, and 49 is some number of sevens, and 56 is one more seven than 49…” He nods his head because he doesn’t know yet where I’ll take this. “Then when you get the answer for 56, the answer for the other is one less. When you solve one, you solve the other.”
This he understands, and he says, “Because 49 and 56 are next to each other on the sevens line.”
So now I ask him what 9×7 is, and everything snaps into place. He sees the pattern behind multiples of seven and gets the answers to the original problems. And he already knows 9x(whatever) because 10-1 is another pattern.
Posted in Make
Interview with Dr. Melissa Kibbe: cognitive psychology of kids ages 4-6 learning algebra
Dr. Melissa M. Kibbe is assistant professor of Psychological and Brain Sciences at Boston University. She received her PhD in Cognitive Psychology from Rutgers University in 2011, after which she completed postdoctoral research at Johns Hopkins University. Dr. Kibbe’s research focuses on how infants, children, and adults represent information about objects (e.g. perceptual features, animacy, group statistics, numerosity, verbal labels). She also studies how infants’ and children’s early understanding of objects and numbers impacts how they learn formal systems, such as mathematics and physics, later in life.
Meeting with Dr. Melissa Kibbe on September 15
We held an open, free online event in our Math Future series on September 15 8 PM Eastern Time, where we met with Dr. Melissa Kibbe to learn the latest research on early algebra: how children ages 4-6 can use their Approximate Number Sense to “solve for x”.
Here is Melissa’s short interview for readers of Moebius Noodles.
Can you share an anecdote or an episode about math from where you were a little girl?
At my elementary school, there was a path from the crosswalk to the school that made a 90-degree angle. But the kids would walk across the grass to take a shorter path, creating a well-worn dirt hypotenuse. Every day, I would vary the path I took, trying to match my speed to the children walking the other paths, and then I would observe who got to the end of the path first. I was trying to figure out the relationship between the legs and the hypotenuse of the right triangle using experimentation. While I didn’t derive the Pythagorean theorem from my daily experiments, I did get an approximate sense of the proportion of the length of the hypotenuse to the lengths of legs. That kind of experimentation helped to learn the formal rules of trigonometry later on.
What made you study 5-year-olds doing algebra, and other young math?
Infants, children, adults, and some animals all have a built-in approximate “number sense”. We use this number sense whenever we want to, say, compare which of two jars has the most jelly beans or when we want to estimate how many people are in a crowd. We asked whether children could harness their approximate number sense to solve the kinds of problems that are usually not introduced much later in formal schooling, and with which children often struggle. That is, we thought that presenting problems in a more intuitive way may help kids “solve for x”. In our studies, children did not have to count or use symbols. In fact, when we did show them problems with digits and operator-signs, they did quite poorly. Rather, they could rely on their approximate “gut sense” of quantities to solve for the hidden addend in our problems.
Can you share some algebra-inspired activity our readers can try with their toddlers or young kids? What are your personal favorites for when you work with kids, and why?
In our studies, we show children problems with unknown addends (like 5+x=17), but we present them in an intuitive way, embedded in a story. Children saw a stuffed animal character, Gator, who had a “magic” cup that will add more objects to a pile of objects, such as buttons or pennies. Children get to see the piles before the cup added its quantity, and after. We showed children the “magic” cup working on different piles, but always adding the same amount every time. Then, we pretended to mix up some cups, and showed children the quantities inside two different “magic” cups, only one of which matched the quantity that Gator’s cup had added; children were asked to choose which cup belonged to Gator. Children were quite good at choosing which quantity was in the animal’s cup, showing that they had an intuitive sense of the quantity of the unknown addend. Parents can try these kinds of activities with children at home, using items that are found around the house and a favorite toy.
Some people say only some demographics can do early algebra – gifted kids, children of STEM professionals, richly supported kids… What do you say to that?
In our studies, children needed only to rely on their gut-sense of number. Since the children were between 4 and 6 years old, only a few of them had been introduced to formal math in school. Our studies suggest that the ability to “solve for x” is present early in development.
Anyone can try this with kids!
Posted in Grow
