Math Stories – Cut and Fold
I remember that when I was little, I derived a sort of enjoyment, a feeling of accomplishment, from doing math worksheets. For better or worse, my son is the exact opposite. Worksheets do nothing, but turn him off math. And so do most of the puzzles. But he loves stories. So a lot of what I do is tell stories.
The first time I told a cut-and-fold story was a long time ago. We were exploring symmetry, playing mirror games and building with blocks before. That first time I folded pieces of paper only 1 or 2 times before cutting while telling a story. My son really loved it, but then we moved on to other things…
But a few weeks ago I read a wonderful post on the Map is Not the Territory blog. In her Scissor Stories post, Malke not just posted the pretty photographs of symmetry art (like the one above), but wrote a detailed script, a story she told to a group of children. While my stories were monologues, she conversed with children, asked them questions, and helped them notice the math in the story. I encourage you to read Malke’s post. It was so inspirational to me, that the very next day I tried our cut-and-fold stories again, this time in a way Malke’s done it.
The story I told my son was about three friends. He immediately made it about him and his two best buddies and how they were ninjas. Each friend was represented by a square of origami paper. We then folded each square, trying to predict what would happen to them after each fold. Then each square ninja had some adventures along the way and made some tough choices. In the end, we tried to predict what each square would look like. Typically, my son does not like guessing, but he was so into the story, that he kept offering his predictions throughout the game. And, unlike his usual answers of “I don’t know” and “A million” and “17 million and twenty five” the guesses he offered were well thought-out and he could usually explain how he arrived at them.
Unlike Malke, I totally forgot to take pictures throughout the game or record the narrative. But honestly, if you read Malke’s post, you will know exactly what to do and will see all the opportunities for improvisation.
I promise that next time I tell math stories to my son, I’ll keep a better record of them. Would you be interested in these stories? Do you tell math stories to your children? Would you like to share them on this blog? If yes, e-mail me at yelena(at)moebiusnoodles.com If you already have your stories posted on your own blog, please share a link in the comments!
Posted in Grow
Newsletter July 30, 2012
Welcome to adventurous math for the playground crowd! I am Moby Snoodles. Please write me – I reply to everyone! moby@moebiusnoodles.com
Book news
We are about half way through illustrating the book: a good time to pause for reflection. If math were a story, its genre would be action/adventure. Have someone convinced you math consists of facts, theorems, diagrams and formulas? They lied. These things – these static objects – are just snapshots of the dynamic experience of math. The real mathematics consists of actions: putting together data to dig up a new fact, postulating a theorem, building a diagram, manipulating a formula.
If this is so, we “Moebius Noodles” authors ask ourselves, why are we making a book rather than a playground, a virtual world, or at least a suite of interactive lapware? There are three reasons, we answer ourselves! First, while books are not adventures, historically they have been excellent social objects for inviting people and communities to have their own adventures. This still holds true circa 2012: we checked. Second, all of us, and our kids, love books of the sort we are making. You may laugh, but we will read and re-read “Moebius Noodles” ourselves when it is out. And third, we like strong challenges, such as the difficult task of evoking real math actions with mere text and pictures.
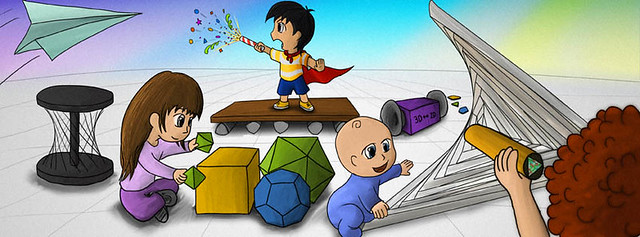
Speaking of challenges: the part of the book dealing with functions is especially action-oriented, so it’s particularly hard to capture in pictures. For example, little kids love doing something over and over again – say, iterating a function machine that doubles. One block, two blocks, four blocks, eight blocks, oh my! How do we show this excitement? Here are some of the many steps from an initial idea to the final version…
An early draft
This is a chain of identical function machines, each doubling the number of cubes. The perspective of the picture – the line of machines coming at you – adds drama and action. Problem: in reality, we only have one function machine, used again and again!
Problem solved?
To address the problem of multiple function machines, how about making a spiral going through the same machine? Check out how much better 3D modeling is for the purpose of showing action, compared to the 2D diagram.
The final version
Many versions later – it’s so hard to show 32 cubes in a row in a meaningful and beautiful way! – here is a peek at the current version of the front piece for the chapter on iterations. Being a static picture, it’s not math, but it’s a very lively portrait of math, if I say so myself. You can feel the action!
Our blogs
Our latest guest blogger is Patrick Honner of the lovely MrHonner.com math appreciation blog. As an excellent high school teacher, Patrick designs many of his activities in ways that provide on-ramps to complex math – from the very ground. This way, however much students have missed up to now, they can still find the current activity meaningful, accessible – and joyful. For Moebius Noodles, Patrick wrote a piece called “Weaving your way through mathematics.” It goes with the part of the book about grids. Here is a picture and a quote.
Once the basic techniques of simple two-color weaving are mastered, more interesting and challenging projects can be undertaken. Using more than one color in an alignment (horizontal or vertical) opens up new patterns, as does using more than two colors. More challenging patterns, tilings, and inversions can be attempted.
Talk to you again July 30th!
Moby Snoodles, aka Dr. Maria Droujkova
Posted in Newsletter
Weaving your way through mathematics
Today’s guest contributor to Moebius Noodles is Patrick Honner, an award-winning high school teacher and a passionate math enthusiast. My favorite part of Patrick’s beautiful blog is the Math Appreciation section, because you can adapt most ideas there for young kids. When I was four or five, I spent a lot of hours weaving with paper. I believe it helped me fall in love with mathematics.
Before starting on Patrick’s game, check out his TED talk on creativity and mathematics! – MariaD
Weaving is a fun and creative way to explore real mathematical ideas. Simple “mat” weaving offers a way to experience basic concepts in geometry and number theory, while encouraging the development of representation and modeling techniques– fundamental mathematical skills.
With some colored construction paper cut into long, thin strips, and some glue or tape, you can get weaving right away! Here are a few introductory activities. More examples and ideas can be found at my website: http://www.MrHonner.com/weaving/
A good place to start is the checkerboard pattern. It is simple, intuitive, and helpful in developing facility with the basic techniques of weaving. Start with two sets of strips of different color; align all of one color horizontally and all of the other color vertically. Now, a simple alternating over-under weave will create the checkerboard.
A more challenging activity with just two colors, each aligned horizontal and vertically, is to weave a tiling of the plane.
This activity definitely requires some planning. Once a type of “tile” is chosen, the weaver must figure out what kind of weaving pattern will produce the desired tilling of the plane. The orange-and-black weave above uses a “short L”-shaped tile and an alternating, 1-over / 2-under pattern. The orange-and-purple weave uses a “long L”-shaped tile and a similar 1-over / 3-under pattern.
Here’s where modeling and representation come into the process. With a blank grid, one can plan out the weave ahead of time, hopefully figuring out what kind of weaving pattern will produce the desired mat. A standard modeling approach can be used, or the weaver can develop their own representation—in both cases, the important mathematical skill of modeling is being developed. Here are some examples of different approaches to modeling various weaves.
Through trial (and error!), the weaver can refine their modeling process and their plans to produce the desired weave.
Once the basic techniques of simple two-color weaving are mastered, more interesting and challenging projects can be undertaken. Using more than one color in an alignment (horizontal or vertical) opens up new patterns, as does using more than two colors. More challenging patterns, tilings, and inversions can be attempted. Here are some examples.
A fun mathematical follow-up to introductory weaving is to consider the question “Which kinds of patterns are weavable?” For example, the following two mats weren’t really woven—some pieces were cut out and taped over other pieces. An interesting and highly mathematical question is, “Would it be possible to produce these mats through weaving alone?”
With some basic supplies and a few simple techniques, significant mathematical ideas can be explored through weaving. And once you’ve mastered the basics, you can start investigating circular weavings, hat and basket weaving, and even try your hands at mathematical knitting!
For more ideas, visit www.MrHonner.com
Posted in Make
Newsletter July 15, 2012
Welcome to adventurous math for the playground crowd! I am Moby Snoodles, and I love to receive mail at moby@moebiusnoodles.com
Paul Salomon from Math Munch, a weekly digest of the mathematical internet, writes:
The Moebius Noodles newsletter was fantastic! I’m expecting my first child in November, a girl. Seeing how well you understand math, I am really excited to have Moebius Noodles on my side as I try to help this girl love life. Thanks so much!
Happy news and glad words, Paul! I bet you will have some good baby math games to share very soon, too!
Book news
One of my favorite aspects of making the book is the transformation of ideas. It all starts from experience design. Then we play with kids, and the designs change – a lot! The ideas become alive. Next, we express these lively ideas in text – and texts are, in turn, represented as pictures. Illustrations, just like texts, go through many drafts and changes. Here is an example from the chapter about iterations of function machines.
November 1998
The cover of the Humanistic Mathematics Network Journal, featuring iteration of a function machine that halves numbers or objects, from the article on young math by Maria Droujkova.
January 2003
Children intent on working with a function machine that doubles the number of toy frogs.
July 2012
Two drafts of the “blueprint” page from the “Moebius Noodles” chapter about iterations. We changed from counters to cookies because you can – at least theoretically – break cookies into smaller and smaller pieces, forever.
Our blogs
Kids Consult is an amazing consulting company founded by three girls, who are just a couple of years older than the target audience of the “Moebius Noodles” book. They review mathematics games, puzzles, books and other materials. We asked them to review three chapters from the “Moebius Noodles” book. Check out the full review on our blog!
Function Machine
- We love the illustration at the top of this page. It really adds reality to the concept.
- Even though this is the most “mathy” of the set, it’s also the most interesting. This is probably because it has no pre-prescribed instructions. Even though the others do need directions and examples, try not to get too constrictive – some parents might think that these are the only variations for the activities. Be sure to mention that those are only possibilities.
Yelena and her son had a chat about signs and symbols – a central young math topic that is likely to play a large role in the next book we are preparing to write. Here is a part of their conversation; read the rest at our blog. I love how Yelena casually weaves a math idea of combining symbols into her son’s play.
Son: Mama, when my tree house is finished, I’m going to invite all my friends and put a big sign “no girls allowed”
Mom: How would you make a sign like that?
Son: Easy, I’ll just make a big red circle with a thick line across like that (draws in the air) and there will be a girl on it, like on bathroom doors.
Mom: Ok, but what if your cousin A comes to visit? Can she play in your tree house? (My son loves playing with his oldest cousin)
Son: (after some thinking about it) Sure!
Mom: But then you need to make a different sign. What would it look like?
Son: (after some more thinking) Ok, I’ll just put her picture next to the other sign. It has to be a smiling picture.
Lapware
Are math snakes in the picture moving or are they not?! Most people will see the motion if they keep focusing on different parts of the picture. Show this gem to your kids, for a beautiful “math minute” together. This mathemagical optical illusion, shared by our friend and supporter Science Jim, is an example of a fractal – that is, a self-similar picture. Self-similarity is deeply meaningful for young kids. It is one of the key topics in the “Moebius Noodles” book.
Talk to you again July 30th!
Moby Snoodles, aka Dr. Maria Droujkova
Posted in Newsletter
