Play Power
This post originally appeared on my personal blog on May 21, 2012. At the end of this post you will find a question related to the following story–I would love to hear your thoughts, ideas and responses. –Malke
______________________
Although I know it already, it never ceases to surprise me just how true it is.
Learners of all ages need the opportunity to experiment with a new medium before putting it to its more formal or expected use. Often this kind of activity is called ‘playing around’ which I have often perceive as a derogatory term in relation to learning. But, in my experience, if you observe children at play long enough and really pay attention you will be astounded by the myriad of ways they are representing their knowledge, understanding and mastery of a subject. Play and exploration are not wasted time. In fact, I think it is exactly the kind of activity that builds the foundation of real understanding.
Here is a case in point from a Math by Design Family Night. Having finally found success using straws and pipe cleaners as a math toy and building material with my own six year old, I decided to include it in the Family Night for the first time. I made some models of polygons and polyhedra, gave the station volunteer a quick orientation, and left the materials to be discovered.
Immediately, it was the most popular station of the eight offered that night. As the children descended, the adults followed, providing lots of helpful advice and some modeling…
…which the kids politely and assiduously ignored as they confidently forged ahead.
This initial inclination to explore the materials on their own terms was fortified by the fact that this was not officially ‘school time’. There was no pressure to do things ‘right’, or follow the rules, or learn and use proper technique.
As a result, most kids cheerfully ignored the formula for folding a pipe cleaner in half and making a nice right angle before inserting it as a connector between two straws, and instead found their own twisty or unequal ways to make it work.
Most also ignored the nice models I had made and created their own. They were having a grand time ‘playing around’ when I noticed something amazing happening. After a very focused exploration period, they started discovering the rules on their own!
This little one, two years old according to her brother who sat beside her, had been methodically putting pipe cleaners into the straws, one after another. It looked like a little gallery of Q-tips, someone joked.
She was working on her own. She must have been at it for thirty minutes and then…she started connecting straws together!
Voila! A hexagon. No one, I suspect, expected much out of a girl so young. And yet, there she was discovering the materials and watching others around her, ultimately creating something for herself. I’d wager that if someone had insisted on sitting her down and showing her how to make a hexagon, she might have been less interested, engaged, focused and, ultimately, successful. Children much older also experienced this same progression.
Throughout the evening, kids kept coming up to me wanting to know if they could have the dodecahedron I made as model for the night. Sort of like a door prize? I said, “Well, no, that one’s mine. But you could make your own!”
Only one girl decided to make one for herself; she also really wanted me to sit next to her while she figured it out. I provided moral support for about five minutes, and then had to ‘go do something…’ A few minutes later, she came and found me with a question and, still later, enlisted support from another adult so she could finally finish it. But you know what? She did all the work, she just needed help ‘seeing’ the structure and pattern. If we had had more time she and I could have talked how to make all the angles congruent so it would be more regular but, still…what a prize!
So, what kind of learning was happening during all this ‘playing’?
I heard a teacher mention that this activity reinforced the learning they were doing in class about corners and sides. Yes, and so much more.
The side of the shape becomes a shared edge. You only need one straw for each edge. The more you build on to your initial shape, the more this aspect of intersection and sharing is apparent. A vertex can be created from the intersection of two, three, sometimes even five different lines/edges.
Depending on what polygon or polyhedron you’re making, the pipe cleaners need to be bent at different angles. An equilateral triangle’s angles are different from a square’s which are different yet again when you create a hexagon, or a pentagon. These are properties you might not truly understand unless you had to make them yourself. And, when every angle in a shape has to be the same, and you’re the one who has to make them that way, you truly build a new understanding of ‘sameness’.
All in all, a good evening’s work. I think my new definition of success is when my project idea is just the starting point and, over the course of the ‘lesson’ not only do multiple right answers emerge but the children are satisfied with their efforts. If the resulting mess is any indication, I’d say it was an entirely satisfying evening.
You can answer the question “How do you transition from playing math to more formal math activities/lessons?” here or on the Moebius Noodles Question & Answer Hub. Thank you in advance for sharing your thoughts and ideas!
__________________________________
Malke Rosenfeld is a percussive dance teaching artist who blogs about her experiences at the intersection of math, the arts, and learning at The Map is Not the Territory.
Posted in Grow
“Adventurous Math” on Kindle; what is multiplication? Newsletter February 17
Subscribe to Moebius Noodles newsletters
Pinterest | Twitter | Facebook | Google+
I am Moby Snoodles, and this is my newsletter. Send me your requests, questions and comments at moby@moebiusnoodles.com

Book news
Adventurous Math for the Playground Crowd is now available for Kindle and other e-readers in the .moby format.

What is multiplication?
Questions, comments, ideas, blog posts about multiplication are coming in for the project that starts with our online and local Math Cafe on February 18th. @twen1977 and quite a few others ask about memorization:
Is there a better way to learn multiplication tables besides just memorizing?
Yes, yes there is! We will be addressing this and other parent and teacher questions during the Math Cafe, and throughout the multiplication project. Meanwhile, head for the blog to check out the variety of posts that bridge multiplication and other human endeavors.
Marina Mersenne presents an advanced theater game, The Mirror Routine – easy enough for toddlers to learn, complex enough for professionals to use. Of course, symmetry is one of the major models of multiplication.

Malke Rosenfeld invites you to share memories of Beautiful Objects from your childhood, using a hands-on manipulative about group theory (hence multiplication!) as her example. Alexander Bogomolny, a mathematician and math educator of Cut-the-Knot fame, responds with a touching example:
Puddles, I was absolutely taken with the puddles. The surprising thing was that an object’s reflection moved when I moved while it was not mine but the object’s.

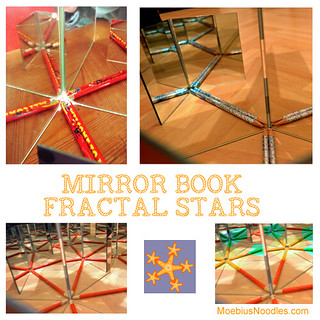
Yelena McManaman shares an invention of her son Mark (7), who found a way to model fractal stars with mirror books: a combination of two models of multiplication in one beautiful project.
Mark Gonyea gave us an interview about his artistic 100-charts, with many cells built on arrays, fractals, symmetry and other multiplication patterns.

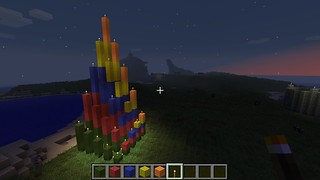
The Natural Math crew added new finds to our big history of the Multiplication Tower, a slightly mysterious 3D-modeling project that people have been independently reinventing since the 1970s.

In response to examples made of wood, LEGO, beads and more, we got two fresh versions. The history continues to grow: after our story came out, Jenny’s son Viktor built his multiplication towers in Minecraft, and Sheryl Morris remixed the bead version in Montessori colors.

Sharing
You are welcome to share the contents of this newsletter online or in print.
Talk to you in two weeks! Moby Snoodles, aka Dr. Maria Droujkova
Posted in Newsletter
Mirror book fractal stars
Yelena McManaman’s son Mark loves deep ideas. Yelena writes: “Mark is obsessed with the idea of symmetry and infinity. Now he’s also trying to build up on his “zero infinity and infinite zero” ideas.” In one of his latest experiments, Mark invented a way to model fractals with two mirror books.
There is a chapter on fractals and another chapter on mirrors in our Adventurous Math book, but I’ve never thought of combining the two ideas! This is a great example of why we need children in our workplaces and creative projects. Young kids are great at divergent thinking, unexpected connection, and noticing details. Imagine the worlds where engineers and scientists developed a way to consult Mark and other young kids during explorations.
Here is Mark’s fractal star, with four rays made out of double pencils. Note how both mirror books are opened at 90 degree angles, to make four copies.

After Yelena sent me this cool photo, I just had to make my own mirror book fractal stars! I played with three-ray stars and five-ray stars.


When kids draw fractals by hand, they often use new color for each new level. I colored levels of my five-ray fractal in Photoshop: the first level stayed the original orange, the second level is yellow, and the third level is green.

This is how the setup with two mirror books looks from the top. You can only see a few levels of the fractal from that angle:

You can get small mirrors in craft stores, and large mirror tiles in home improvement and hardware stores. Invite your kids to join Mark in his experiments! If you want to share your mirror book or other fractal creations, email me your photos – moby@moebiusnoodles.com

Posted in Make
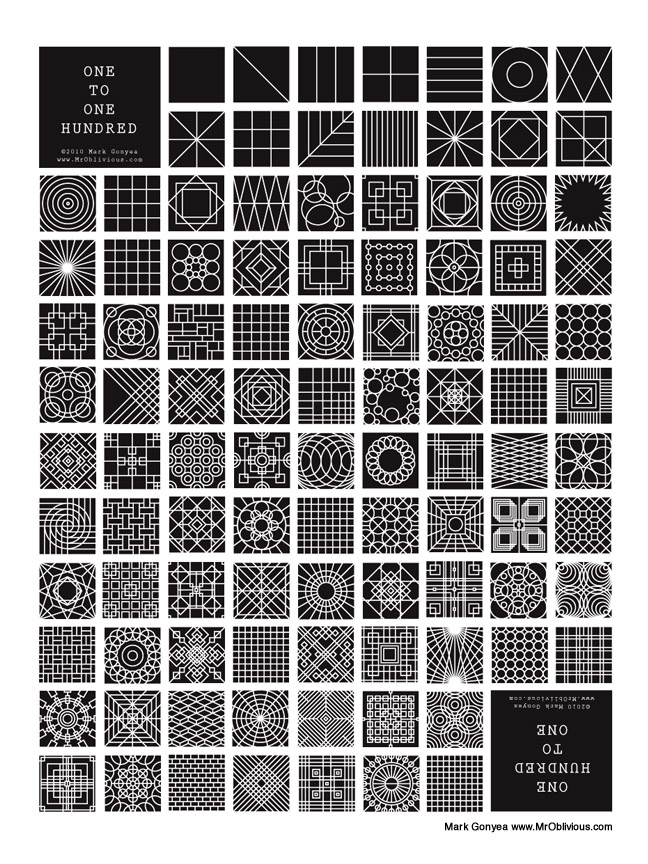
One to one hundred: a series of posters from Mark Gonyea
Mark Gonyea is an artist and graphic designer who recently crowd-funded an inventive and elegant series of 100-charts. The artwork caught my eye because the patterns were both adventurous and algebraic. Want to borrow my math goggles? Go on a scavenger hunt within the posters to find these mathematical structures:
- Arrays
- Star sheafs and star polygons
- Fractals
- Tessellations
- Mirror and radial symmetry
- Randomness
My finds (and the brief definitions of these math terms) are at the end of the post. What else do you see in the posters?
I sent Mark a few interview questions.
— Do you remember how math was for you when you were a little boy?
— I remember being good at math. I liked Algebra the most, which when I think about it is not unlike graphic design to me. It’s adding, subtracting, dividing and multiplying. It’s all about trying to solve a problem.
— What feelings drove you to create that many complex hundred-charts? What’s the story of this project, and the meaning of the story for you?
— I started creating multi-panel posters around the year 2000 as a reason to exhibit at the San Diego Comic-con. So at first, they were very much narrative and kind of a long comic strip in nature. Fairly quickly I started to do more design focused posters as well and a few years ago one of those posters was reprinted in an art book about abstract comics. The piece was a 48 panel comic called Squares in Squares where each panel pulled back to reveal more and more squares surrounding each other with no narrative other than the increasing complexity of shapes. So when I started to think about what kind of Kickstarter project I wanted to do I thought back to the popularity of that poster and it really got me thinking of doing a series of sequential designs.
— Can you give a few design tips for families and Math Circles creating their own artistic number charts?
— Definitely start small. I realized pretty quickly how complicated things can get, even before I got to the larger numbers. With the first 1 to 100 poster (Shapes) I think it was actually #14 where I realized “Oh, this is going to be more involved than I thought” and that number became my first asymmetrical panel.
— Where can we find your work online?
— MarkGonyea.com is the best place to see what I’m up to and where to find my work online. Thanks!
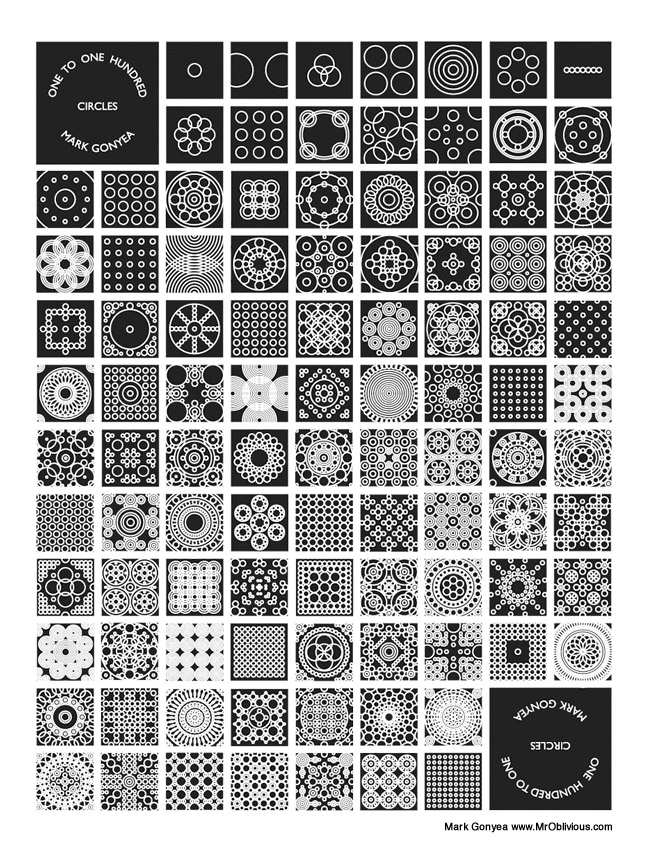
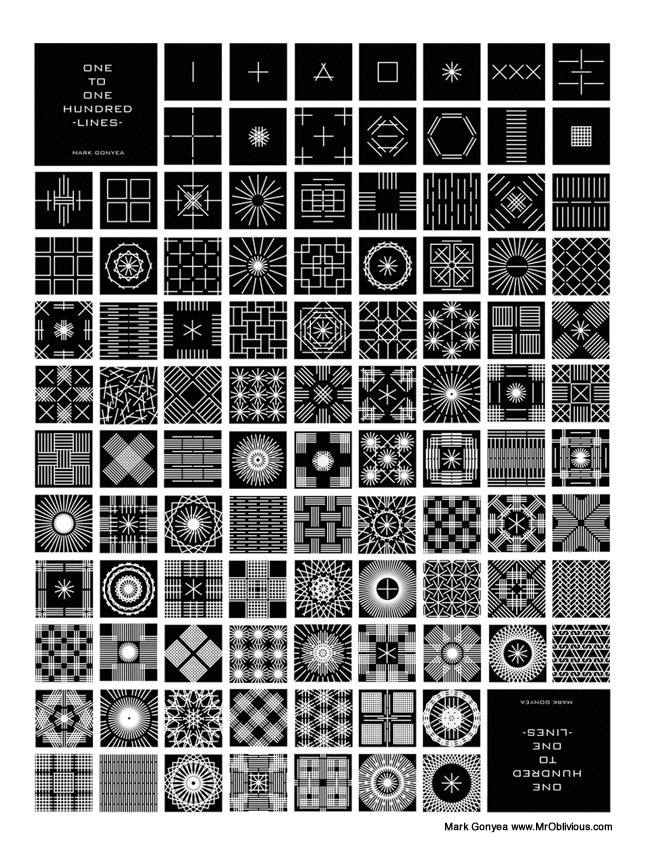
Here are examples I found. It strikes me that many of them have to do with multiplicative reasoning: grids, symmetry, and fractals for sure! What other math ideas do you see here?

Posted in Make



